Tetrahedron

format_list_bulleted Contenido keyboard_arrow_down

ImprimirCitar

3D model of a regular tetrahedron

A tetrahedron (from the Greek τέτταρες 'four' and ἕδρα 'seat, base or face') or triangular pyramid is a polyhedron with four faces, six edges, and four vertices. The faces of a tetrahedron are triangles and three faces meet at each vertex; If the four faces of the tetrahedron are equilateral triangles, equal to each other, the tetrahedron is called regular. It is also a Platonic solid. Otherwise a tetrahedron is a pyramid with a triangular base.

The tetrahedron is the simplest of all ordinary convex polyhedra and the only one with fewer than five faces.

Other elements

In addition to face, vertex, edge, and height, other elements are required:

Inscribed sphere

It is the sphere whose center is inside and the sphere is tangent to all four faces. Its radius is usually denoted by r.^{[citation needed]}

Circulated area

This is the name given to a sphere such that the vertices of the tetrahedron are on the spherical surface and its center is an interior point. Its radius is usually denoted by R.^{[citation needed]}

Bimediana

It is the segment that joins the midpoints of two opposite edges; is denoted by m_i, i = 1,...,4. The one with the shortest length is written m.

Geometric properties

Not regular tetraedro.

In every tetrahedron the following properties are verified:

The segments that unite the midpoints of opposite edges (bimedians) are concurrent at a point; this is the midpoint of such segments.
The segments that unite each vertex with the intersection points of the medians of their opposite face are also concurrent in a point, which divides them by separating three quarters from the side of the respective vertex (Commandinal Theorem).
The six planes perpendicular to the edges by their midpoints pass by the same point, center of the sphere circumscribed to the tetraedro.
Perpendicular straights to the faces by their circumcentre are concurrent at a point, center of the sphere circumscribed to the tetrahedron.
The bisector planes of the inner dieros of a tetrahedron converge in a equidistant point (incenter) of the four faces, center of the sphere inscribed to the tetrahedron.
The plane that passes by an edge and by the middle point of the opposite edge biseca the regular tetraedro in two congruent figures. The volume of each part is half the volume of the original figure.
The tetrahedron has characteristic of Euler 2.
The bimedians are cut into an inner point called mass centerAnd they're biking each other.

Metric properties

Volume

File:Triangle_GeometryArea.svg

Calculation of the area of a triangle or area of a tetrahedron face:

{displaystyle A={tfrac {bcdot h}{2}}}

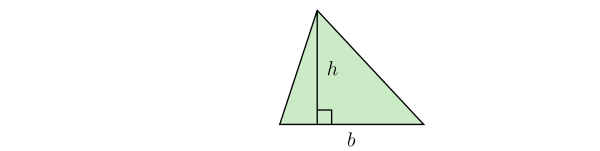
The volume of a tetrahedron is

V =

{displaystyle {frac {1}{3}}times Btimes h}

, being B the area of a face and h the height of the tetraedro, that is the perpendicular segment to the base plane from the opposite vertex.

The volume of a height tetrahedron $h$ and regular base side $L$ That's it.

{displaystyle V=hcdot L^{2}cdot {frac {sqrt {3}}{12}}}

Analytical geometry of space resource

There is a general formula to calculate the volume of a tetrahedron OABC, where O coincides with the origin of coordinates, whether regular or not, based on Cartesian coordinates (x, y, z) of three of its vertices, A, B, C:

$V=frac{1}{6}, begin{vmatrix} x_A & x_B & x_C \ y_A & y_B & y_C \ z_A & z_B & z_C end{vmatrix}$

This formula can also be written in terms of the Cartesian coordinates of the four vertices $scriptstyle {(x_1,y_1,z_1), (x_2,y_2,z_2), (x_2,y_2,z_3) (x_4,y_4,z_4)}$ ; the volume of a tetrahedron (regular or not) is given by the following formula:

$V = frac{1}{3!}, begin{vmatrix} x_1 & y_1 & z_1 & 1 \ x_2 & y_2 & z_2 & 1 \ x_3 & y_3 & z_3 & 1 \ x_4 & y_4 & z_4 & 1 end{vmatrix}$

Another formula, which can be obtained from the previous one, allows to calculate the volume of a tetraedro, regular or irregular, knowing the length of two opposite edges $l_1$ and $l_2$ , distance $h$ and angle $theta$ among them:

$V=frac{1}{6} cdot l_1 cdot l_2 cdot h cdot sin theta$

This formula is applicable to calculate, approximately, the volume of a embankment, of a road or a dam of loose materials, for example, from the length of its coronation $l_1$ , the length at the base $l_2$ and its height $h$ .

The generalization of Heron's formula allows us to calculate the volume of a tetrahedron from the measure of its edges a, b, c, ab, ac and bc:

${displaystyle V={frac {1}{6}}{sqrt {,{begin{vmatrix}ucdot u&ucdot v&ucdot w\vcdot u&vcdot v&vcdot w\wcdot u&wcdot v&wcdot wend{vmatrix}}}}={frac {1}{6}}{sqrt {,{begin{vmatrix}a^{2}&{frac {a^{2}+b^{2}-ab^{2}}{2}}&{frac {a^{2}+c^{2}-ac^{2}}{2}}\{frac {a^{2}+b^{2}-ab^{2}}{2}}&b^{2}&{frac {b^{2}+c^{2}-bc^{2}}{2}}\{frac {a^{2}+c^{2}-ac^{2}}{2}}&{frac {b^{2}+c^{2}-bc^{2}}{2}}&c^{2}end{vmatrix}}}}}$

u, v and w are the vectors determined by the edges a, b and c. The formula is taken into account ${displaystyle (u-v)cdot (u-v)=ucdot u+vcdot v-2ucdot v}$ .

Heights of the tetrahedron

A tetrahedronnot necessarily regular) is defined in R³ knowing the coordinates of their four vertices, for example $scriptstyle V_1 = (x_1, y_1, z_1), V_2 = (x_2, y_2, z_2), V_3 = (x_3, y_3, z_3), V_4 = (x_4, y_4, z_4)$ . Any of its four faces is defined by the triangle formed by the three vertices of it, each of the faces defines a plane (flat by three points) base of the height that forms with the opposite vertex, being said opposite vertice the remaining point that was not used when defining the face. You can imagine a tetraedro thinking that his base is defined by the triangle formed by three vertices any of the same to which we will call $scriptstyle V_2, V_3$ and $scriptstyle V_4$ and that there is a vertice opposite to that base we shall call $scriptstyle V_1$ .

To calculate the height that forms an opposite vertex any with its base face you only have to put the values of that opposite vertex in $scriptstyle V_1 = (x_1, y_1, z_1)$ and then put the values of the three vertices of the opposite face to the same $scriptstyle V_2 = (x_2, y_2, z_2), V_3 = (x_3, y_3, z_3)$ and $scriptstyle V_4 = (x_4, y_4, z_4)$ then apply them in the following formula:

${displaystyle {text{Altura}}=left|{frac {x_{1}(y_{2}(z_{3}-z_{4})+y_{3}(z_{4}-z_{2})+y_{4}(z_{2}-z_{3}))+{y_{1}}(x_{2}(z_{4}-z_{3})+x_{3}(z_{2}-z_{4})+x_{4}(z_{3}-z_{2}))+{z_{1}}(x_{2}(y_{3}-y_{4})+x_{3}(y_{4}-y_{2})+x_{4}(y_{2}-y_{3}))-x_{2}y_{3}z_{4}+x_{2}y_{4}z_{3}+x_{3}y_{2}z_{4}-x_{3}y_{4}z_{2}-x_{4}y_{2}z_{3}+x_{4}y_{3}z_{2}}{sqrt {(-x_{2}y_{3}+x_{2}y_{4}+x_{3}y_{2}-x_{3}y_{4}-x_{4}y_{2}+x_{4}y_{3})^{2}+(-x_{2}z_{3}+x_{2}z_{4}+x_{3}z_{2}-x_{3}z_{4}-x_{4}z_{2}+x_{4}z_{3})^{2}+(-y_{2}z_{3}+y_{2}z_{4}+y_{3}z_{2}-y_{3}z_{4}-y_{4}z_{2}+y_{4}z_{3})^{2}}}}right|.}$

To know the four heights of the tetrahedron, it is enough to rotate the coordinates of its vertices. This formula does not require that the tetrahedron be regular, it is valid for any non-degenerate tetrahedron.

Regular tetrahedron

It is a polyhedron formed by four faces that are equilateral triangles, and four vertices in each of which three faces meet. It is one of the five perfect polyhedra called the Platonic solids. It is also one of the eight convex polyhedra called deltahedra. Applying the standard nomenclature of Johnson's solids, it could be called a triangular pyramid.

is the Geometric expression that represents the number of arist and vertices faces that the regular tetraedro has. It has in the left superscript six (6) uniform edges of flat category, the numeredron indicates four faces even (4) and the basic polygon (3) indicate that this polyhedron has triangular faces congruent equilateral. When we interpret the numbers placed in the polyhedral pair 4(3, 3) that symbolizes the tetrahedron, we read that there are four uniform vertices and that each vertices bind three uniform equilateral triangles. ]]

For the Pythagorean school, the tetrahedron represented the element of fire, since they thought that the particles (atoms) of fire had this shape.

Various measurements using the edge

Exclusively from the edge a the rest of the fundamental dimensions of a regular tetrahedron can be calculated. Thus, for the singular spheres of the tetrahedron:

Radio R of the sphere circumscribed to the tetrahedron (which contains on its surface the four vertices of the same):

R= frac{ sqrt{6} }{4} cdot a approx 0,6124 cdot a

Radio r of the sphere inscribed to the tetrahedron (the tangent to the four faces of the tetrahedron):

r=frac{ sqrt{6} }{12} cdot a approx 0,2041 cdot a

Radio ρ from the tangent sphere to the six edges of the tetrahedron:

rho = frac{ sqrt{2} }{4} cdot a approx 0,3536 cdot a

In a regular tetrahedron, each pair of opposite edges (those that do not meet at the same vertex) are orthogonal to each other, the minimum distance between them being the segment that joins their midpoints, of double length to the radius ρ of the sphere tangent to the edges of the tetrahedron.

The height H of the regular tetrahedron (supported the tetrahedron in a stable way on a horizontal plane, perpendicular distance from the support plane to the opposite vertex):

{displaystyle H={frac {sqrt {6}}{3}}cdot aapprox 0,81649658909cdot a}

Volume, area and development

Animation of one of the developments of the tetrahedron.

Given a regular tetrahedron with edge a, we can calculate its volume V using the following formula:

${displaystyle V={frac {sqrt {2}}{12}}cdot a^{3}approx 0,1178511302cdot a^{3}}$

And the total area of its faces A (which is 4 times the area of one of them, A_c), by:

${displaystyle A=4cdot A_{c}=4cdot {frac {sqrt {3}}{4}}cdot a^{2}={sqrt {3}}cdot a^{2}approx 1,732050808cdot a^{2}}$

Angles

The plane angles formed by the concurrent edges are, as in the rest of the Platonic solids, all equal; and with a value of 60º (π/3 rad), when constituting the interior angles of an equilateral triangle.

The dihedral angles formed by the faces are, as in the rest of the Platonic solids, all equal, and can be calculated:

delta=2 cdot arcsin {frac{sqrt{3}}{3}} approx 1,23 text{rad} (70^circ 31' 43,61'')

The solid angles formed by the vertices are, as in the rest of the Platonic solids, all equal, and can be calculated:

$omega = frac {A_c} {H^2} = frac {frac{sqrt{3}}{4} cdot a^2}{left(frac{sqrt{6}}{3} cdot a right)^2} = frac{3sqrt{3}}{8}sr approx 0,650 text{sr}$

Particular properties

Symmetry

Rotations around an axis and reflection on a plane of a regular tetrahedron.

A regular tetrahedron has four axes of symmetry of order three, the lines perpendicular to each face through the opposite vertex of the tetrahedron; and six planes of symmetry, those formed by each edge and the midpoint of the opposite edge. This makes this field have a total symmetry order of 24: 2x(4x3).

The above symmetry elements define one of the tetrahedral symmetry groups, the so-called T_d according to Schläfli notation.

The tetrahedron also has three axes of symmetry of order two: the lines that pass through the midpoint of one edge and through that of the opposite edge.

A plane that passes through an edge and the midpoint of the opposite edge of a regular tetrahedron is a plane of regular tetrahedron symmetry. In total there are 6 planes of symmetry.

Conjugation

The regular tetrahedron is the only Platonic solid conjugate of itself (usually called self-conjugate), since the conjugate polyhedron of a tetrahedron with edge a is another tetrahedron with edge b, such that:

{displaystyle b={frac {a}{3}}}

Projections

The orthogonal projections of a regular tetrahedron on a plane can be:

Triangles;
- In particular, if the projection plane is parallel to a face, the tetrahedron projection is an equilateral triangle, corresponding to a face in real magnitude.
Quadrels;
- In particular, if the projection plane is parallel to two opposite edges of the tetrahedron, the projection is a square, with one side equal to the length of the tetrahedron edge divided by the square root of two.

Sections

Cross-section.

The infinitely many sections that we can take from a regular tetrahedron can result in:

Triangles;
- In particular, any section taken by a plane parallel to one of the faces of the tetraedro is an equilateral triangle.
Quadrels;
- In particular, any section taken by a plane parallel to two opposite edges is a rectangle.
- If, in addition to being parallel to two opposite edges, the equidistant cut plane of both, the resulting section is a square side half of the tetraedro edge. As there are three pairs of opposite edges, a regular tetrahedron can be split in this way by three different planes.

Composition, decomposition and twinning

It is possible to include a regular tetrahedron in a cube in such a way that each of the vertices of the tetrahedron coincides with a vertex of the cube, the edges of the tetrahedron coinciding with diagonals of the faces of the cube. The volume of the cube required to enclose a tetrahedron in the manner described is three times that of the tetrahedron. There are two possible positions to include the tetrahedrons in the cube in this way;

The edges of the tetraedros placed in both positions are perpendicular to each other (they are the cross diagonals of the faces of the cube).

The three square sections of both tetraedros match.

The solid set (or mass) of both is a compound polyhedron called Kepler's octangle star (stella octangula).

The common solid of both is a regular octahedron of half edge than that of tetrahedrons.

It is not possible to fill the space only with regular tetrahedra (although, it seems, that Aristotle believed so), but it is possible to do it with elements formed by a combination of a regular octahedron and two regular tetrahedrons.

Of the infinitely many ways to truncate a regular tetrahedron, there are two that produce unique results:

Trimming the tetrahedron with planes that pass through the middle point of their edges, we get a regular octahedron.

Trimming the tetrahedron with planes that pass through the third part of its edges, we get a solid arquimedian that takes the generic name of truncated tetrahedron.

A tetrahedron cannot be stellate, since all intersections between the planes of the tetrahedron's faces are edges of the tetrahedron.

Tetrahedrons in nature and technology

Thermal structure of methane. The C-H links are directed towards the vertices of a regular tetrahedron.

The tetrahedral form occurs naturally in certain covalently bonded molecules. The most common of these is the methane molecule (CH₄), in which the four hydrogen atoms are located approximately at the four vertices of a regular tetrahedron of which the carbon atom is the center..

There are also natural crystalline structures with a tetrahedral shape.

Despite the tetrahedron being a polyhedron with a simple and totally regular shape, there are not many objects in common use based on its shape.

As a storage medium it is a disastrous shape: it is not possible to fill the space with it, which would be the way to not waste volume between the pieces; nor is it easily stackable as it does not have parallel faces; and, furthermore, it is very inefficient: to contain a liter of product, more than 7.2 dm² of "wall" are necessary, while using a cube with 6 dm² is sufficient. Despite all these drawbacks, the Swedish company Tetra Pak developed a tetrahedral-shaped metallised carton pack in the 1950s, solely because it was remarkably simple to manufacture: simply roll a sheet of paper into a cylinder, then flatten its ends. two ends, but in perpendicular directions, thus achieving a tetrahedron.

In any position that a tetrahedron is supported, one of its vertices is vertical upwards. For this reason, the manufacture of certain models of mobile road marking elements is based on their shape since, since the position in which they rest is indifferent, their placement is quick and simple, and they cannot be knocked down by vehicles.

Trapods for broom.

It is a simple shape with great ease to get stuck and snagged, since its vertices are very sharp and directed in all four directions. For this reason, its shape is sought in elements whose main function is to hook, such as ship anchors (in outline, an anchor is formed by the two opposite edges of a tetrahedron joined by their perpendicular), or to lock together, such as breakwaters. reinforced concrete for defense against waves. There are at least three frequently used models based on the shape of a regular tetrahedron:

The tetrapodes, formed by four cone trunks placed according to the heights of a regular tetraedro, between its vertices and its center.

Scumbags.

The doloses (plural dolos), designed by the engineer Eric M. Merrifield, formed by three straight pieces, two materializing the opposite edges of a regular tetrahedron and a third uniting them for their perpendicular.
The akmon (yunque)developed at the Delf Hydraulic Laboratory (Netherlands), similar to the doloses, but more robust.

At the beginning of the 20th century Alexander Graham Bell, inventor of the telephone, experimented extensively with kites, in order to develop manned flight with heavier-than-air vehicles, and arrived at this form after a series of experiments.

Tetrahedral kites are composed of multiple cells in the shape of a tetrahedron, in which only two of its faces materialize. He came to build huge kites, made up of a large number of these cells.

Done for role play.

In 1907, he built one with 3,393 cells that he dragged with a steamboat, being able to lift it 50 m with a crew member on board. He later tried other even larger constructions, and equipped with motors, but they did not give the desired result. The engines lacked power and the constructions were excessively fragile, so he abandoned the project, devoting himself to other activities.

NASA's Mars Pathfinder space probe was also shaped like a tetrahedron, whose faces opened like petals upon landing on July 4, 1997, to allow the Sojourner robot inside it to escape.

Another practical application of the tetrahedron is to shape the four-sided die, whose written notation is "d4" and which is used above all in many role-playing games. As this die does not show one face up, it usually has the value of the roll marked on the vertices or on the base.

Related polyhedrons

Pyramids
I mean,	Triangular	Square	Pentagonal	Hexagonal	Heptagonal	Octogonal	Eneagonal	Decagonal...
Improper	Regular	Equilateral		You know what?

Contenido relacionado

Más resultados...