Slide rule
The slide rule is a calculating instrument that acts like an analog computer. It has several mobile numerical scales that facilitate the quick and comfortable performance of complex arithmetic operations, such as multiplication, division, etc. Its scales have been modified in order to be adapted to specific fields of use, such as civil engineering, electronics, construction, aeronautics and aerospace, finance, etc. The most common scale is around 25 cm in length (10 inches) that reaches a precision of three significant figures, there are "pocket" with minor precisions that reach approximately 10 cm. Its historical evolution had a peak that coincided with the advent, at the beginning of the last third of the 20th century, of the first electronic calculators and primitive personal computers.
From the middle of the 19th century until its decline in the last third of the 20th century its use was more or less widespread in areas of engineering, administration and pre-industrial crafts. In the first decades of the XX century, its use was so widespread that there was no engineer who did not have access to some slide rule. There were several companies throughout the world that provided various models. The oldest models were made on scales engraved in wood, brass, bone, and later plastic was introduced. In the 1970s their use gradually disappeared, until in the last decades of the XX century there were hardly any generations of engineers who used them. used. Its use has been relegated to museums, organizations of friends, and to specific applications within the basic teaching of mathematics.
History
Devices with scales, used in calculation, have been used by various scientists before the 16th century. It is precisely Galileo Galilei who describes a sector used in the calculation of trigonometry formulas. Some of these primitive calculation systems come from ancient astrolabes, or medieval instruments such as the volvelle, as well as various tools used in navigation, in astronomy such as celestial planispheres, or gnomonic nomograms.
Some historians point out that its inventor was the mathematician Edmund Wingate in the mid-XVI century, while others ascribe its invention to the Reverend William Oughtred in 1636. The notable advance in its development began with John Napier's study of logarithms that he published in 1614. Years later it was the astronomer Edmund Gunter when he applied the idea of logarithms to calculation scales in his canon triangulorum giving rise to the first mathematical applications of the logarithmic scale. Gunter modified the scale so that he could perform trigonometric calculations as well. This instrument was used to perform dead reckoning in navigation and was called the Gunter scale. Gunter made contributions to other mathematical calculating tools. William Oughtred takes Gunter's ladder and decides to put two ladders that slide into each other. By aligning the values of the scales it was possible to perform arithmetic calculations, giving rise to the prototypical view of the first slide rule. Among other 17th century scientists who noticed the use of slide rules is Richard Delamaine who claimed to be its discoverer, disputing its originality with William Oughtred himself. At the end of the XVII century, these calculation rules were used in different versions, and with various applications. Among some of the pioneers of slide rule designs is Robert Bissaker who in 1654 together with Seth Patridge in 1657 already proposed a movable rule in his design.
In 1675, Sir Isaac Newton solved cubic equations using three parallel logarithmic scales, and was also the first to suggest the use of a cursor to facilitate readings. Years later he designed Henry Coggeshall the carpenter's rule in order to facilitate the measurement of lengths, surfaces, and the solidity of timber. After improving the initial design, he republished his work under the title A treatise on measurements with a rule of two feet, sliding into one foot (1682). He released a much-modified version in 1722 titled The Art of Practical Measurement Easily Accomplished by a Ruler of Two Feet Sliding to One Foot. Before 1767, seven revisions had been published. In 1683 the English metrologist Thomas Everard described and made an instrument with scales used in the determination of taxes on barrels of beer and wine.
Modifications made in the XVIII century are aimed at changes in shape in order to improve its precision. In this way the engineers James Boulton and James Watt modify the existing designs in order to improve the existing ones. The physicist Peter Roget invents in 1815 the log-log scale with which he can calculate any square root. In 1831 Victor Amadee Mannheim proposed one of the first scale standardization systems, the so-called Manheim system, this system included a sliding rule (runner) that allowed for improved comfort in carrying out certain calculations.
At the end of the XIX century, many builders spread throughout the world in response to the growing demand from engineering offices that requested a larger volume of calculations. Managing the growing number of machines from the industrial revolution requires a greater number of calculations. In 1885 the German immigrant Eugen Dietzgen created a slide rule company in Chicago. In the same city in 1890 Frederick Post created another company with his own patents. The evolution of the Manheim system led to the need to standardize the scales. In the United States, the three leading brands in the development of slide rules were: Keuffel & Esser, Dietzgen and Post Company.
Birth of the Rietz system
The evolution of rules during the period of the Industrial Revolution was increasing. In the 1870s, Germany had two large slide rule manufacturing companies: Dennert and Pape (builders of Aristo), and Faber (later called Faber-Castell). The engineer Johann Christian Denner associates with Martin Pape in the German city of Hamburg to start the company Dennert & Dad. The earliest rulers were made of mahogany, until the use of laminated celluloid in the early 20th century. Engineer William Cox's designs on the duplex slide rule allow Dennert & Pape begin production in the United States for Keuffel & Esser in New York, right up until 1900 when K&E began its own production.
The revolution of scale systems was unified thanks to Max Rietz in 1902. The scale distribution system, called the Rietz system (also Mannheim) made slide rule builders agree by unifying their representations. This improvement allowed an engineer who learned this system to be able to handle any other rule that had the same Rietz system implemented, allowing solutions to be exchanged and compared. The Rietz system was widely accepted until Alwin Walter in 1934 proposed new changes in what is called the Darmstadt System.
Decline of its use in the mid-20th century
Its heyday lasted more than a century, the period between the second half of the 19th century and the third room of the XX Before the advent of the pocket calculator, it was the most widely used calculation tool in science and engineering. In the middle of the XX century, the production of mahogany slide rules was abandoned, and plastics were used. Some of them are special, as is the case of the aristopal used by the Dennert and Pape (Aristo) brand. In 1930 US Lieutenant Philip Dalton invents a circular slide rule that he calls E6B. The E6B rule allows aeronautical calculations on trajectories during the flight, this rule was in use during the Second World War until the mid-sixties. Some companies decide to merge, a well-known case was the Hemmi Bamboo Slide Rule Manufacturing Company Ltd. (referred to as Hemmi Keisanjaku) based in Japan that associates with the North American Post Company during the occupation building the popular POST Versalog rule (1460). Hemmi rulers from the early 20th century century were made of bamboo wood with laminated cellulose. Hemmi models were highly valued in the period from 1920 to 1976.
The use of slide rules continued to grow through the 1950s and 1960s, even as digital computing devices were gradually being introduced into engineering fields. Slide rules fell into disuse with the popularization of the electronic computer. Some famous companies closed production in the mid-1970s. In engineering, it happened mainly with the appearance on the market of the Hewlett-Packard HP-35 model presented on February 1, 1972. Some brands released highly refined variants, such as the Teledyne Post in 1970. The new generation of engineers of the sixties began to use this type of calculators, together with the increasing use of computers, in the generational transition of the eighties, very few engineers used slide rules in the offices.
By 1980 the production of slide rules in the world had practically ceased, although instruments of this type continue to be manufactured in small quantities for very specific uses in industrial sectors, maritime and air navigation or to attend to a minority market of fans and collectors.
Features
First of all, there is a basic support or body, generally parallelepiped, which has a deep longitudinal groove in its central part, which determines the appearance of two subunits, namely, an upper and a lower strip, narrower. In some models it is effectively two independent pieces, rigidly linked together by clamps located at their ends. Another smaller piece in the form of a ruler slides through the central slot, also called a slide (slide in English).
On the front faces of these pieces is where the various scales are engraved. Sometimes the mobile strip also offers scales on its rear part, for which use it has to be inserted in the opposite way in the basic support or its data can be read through holes made in the rear surface of the body. The back of this is also eventually used to inscribe numerical data of interest or even another complete set of scales; The models thus enlarged are usually called duplex, as opposed to the name simplex applied to those that are only operative on their front face. The body of the duplex models is necessarily two assembled parts. Throughout history there have been some devices with several mobile power strips.
Finally, there is usually a mobile and transparent piece, which covers the entire front surface (or the front and back in the case of duplexes), and which only has a fine reference line, called thread, index or crosshairs, although sometimes there may be some other auxiliary line. This piece is called cursor and it is used to facilitate the alignment and reading of the factors involved in operations, especially when the intervening scales are far apart; in duplex models it is essential to transfer data from one side of the device to the other. The cursors on some rulers act like a magnifying glass to improve the detail of the readings. Starting in the second quarter of the XX century, some more mechanically complicated cursors were made: radially pivoted, articulated, etc., but they were never very popular, having been conceived for very special uses.
Precision
The essentials of the instrument are the numerical scales, some fixed and others mobile, through which the operations are carried out. The precision that can be achieved from a given device depends on the length of these scales, since it is limited by the estimations of values that can be made by whoever uses it, a process inherent to the method and which is called visual interpolation or on view. Rules of very different sizes have been built, which at first might seem arbitrary, but it is not; If the work to be done is delicate, the longest rule possible should be used. For example, to achieve an accuracy of one part in 10,000 the scale must have a length of 12 m (as in the Fuller cylindrical model, manufactured after 1878). The usual sizes do not exceed three significant figures in experienced hands, since the last one will almost always be estimated.
Naturally the above presupposes that the marks of the scales are made with absolute precision on the rulers. This is a reasonable assumption in current copies, particularly those commercially available from the early XX century, where Precise mechanical manufacturing techniques began to be applied, but not at all for the precedents, whose scales were made individually or with poor techniques, so many of them were far from perfect. This was another important reason for the slow spread of its use.
It has been expressed that the limited precision of the slide rule is an advantage and not a disadvantage when it comes to practical applications, since the available data on which the calculation is based do not usually exceed three significant figures. In this way, the sensation of false precision is avoided, which electronic calculators can induce if they are not used prudently.
Forms and materials
Throughout time these scales have varied greatly in nature, size and number and have been organized in many different ways, arranging them on rectangular, circular and cylindrical surfaces. The most common embodiment is the one that uses a flat rectangular tablet, from which it derives its name "ruler". The materials used have depended on the times, places and construction techniques available. They have been made of cardboard and papier-mâché, hard wood (such as boxwood), bamboo, metal (bronze, brass and other metals), various plastic materials, etc.
Typology by shapes
The typology based on the shape of the slide rule is the most common. The arrangement and number of scales gives rise to other typologies. If we look only at the shape, it can be seen that a very high percentage of calculation rules are of the rule type, that is, with a parallelepiped shape. It is the most widespread model in the West, and the one from which more units were produced throughout the XX century. However, there were other types of designs that were used in special cases.
The Circle of Calculation
Commonly called a "circular slide rule," it's the only form of the instrument that deserves specific mention other than the rule, not only because it was invented early on but because it offers some very advantageous features. For now, for the same length of the scales, it has a more compact form than the rule. Mechanically it is more solid and could be more exact, since the movement does not depend more than on the central axis. Also, the result of operations does not "exit" never of the scale, which is normally a closed curve, although there were also some in the form of a spiral. On the other hand, it is a little less intuitive to use, since the precision decreases in the scales that occupy positions more inside the circle and the visual interpolation seems to be somewhat more difficult than in the rule. It has never enjoyed the popularity of this one; many times they were used as a vehicle for advertising promotions.
They have been made in two different basic styles. One of them consists of a pair of concentric circles with a radial, fixed or mobile cursor. The circles must be embedded for proper functioning; otherwise the increase in parallax introduces errors in the calculations. Another consists of a fixed disk, with two independent mobile cursors, but that can be joined. Perhaps it is necessary to consider as belonging to a third type the models that take the form of pocket watches, and even wristwatches, managing the movement of the circles and the cursors by means of external crowns; its prototype was conceived by A. E.M. Boucher in 1876.
Cylindrical slide rules
One of the best known is the Otis King designed by Otis Carter Formby King (1876-1944), who was a London grocer who designed and produced a slide rule with a helical scale engraved on a cylinder. Its initial use was for store-specific calculation. The product was named after him due to the patent he obtained. It was manufactured and marketed by Carbic Ltd. in London from 1922 to 1972.
Management of the calculation rule
The key to being able to use the slide rule well is understanding the nature of its scales. In the case of the basic ones, this does not offer much difficulty, nor does it in the case of the most common ones, especially if they are labeled with the symbols indicated in the table above.
If this is not the case, you need to consult the manual of the specific rule model that you have (which is usually not easy because it is the first thing that is lost from the set)[citation required]. Fortunately, there is now enough information about it on the net, with which perhaps this deficiency can be made up. For example, it may be useful to consult the manual for the Faber-Castell Novo-Biplex 2/83 N model. , which is quite detailed and deals with a rule that had many scales. Manuals in Spanish for many European models, including the one just mentioned, along with other extensive information, can be obtained here.
The other two fundamental skills to have are: practice in reading the values and fixing the decimal point.
Slide rule surfaces are often very congested, in an attempt to provide them with maximum functionality, so it's easy to get confused both when setting initial values and getting the result. In addition to this, its latest figures must be estimated. The applicable remedies to avoid these dangers are: a) pay the necessary attention when operating and b) have a little practice.
Logarithmic scales indicate only the decimal part of numbers, the so-called mantissa. In the case of decimal logarithms, the integer part, called "characteristic", is the exponent of the power of ten corresponding to the data. The logarithm of 5600 is 3.74819 (= exp 10³ + 0.74819) and that of 5.6 is 0.74819 (= exp100 + 0.74819). This is why the scale repeats every ten integers, in what is sometimes called a cycle. The scales C and D, the basic scales of all slide rules, are scales of one cycle, they do not cover more than 1 to 10, but this last 10 is also represented by a 1 because it is the beginning of the next ten. The scales A and B are scales of two cycles, arranged in the same space as the tens of the C and D. That is why their values represent the squares of these and so on. But that means that you have to be careful not to confuse the first ten with the second, nor the first digits of the numbers with the second. For example, 1.5² is 2.25, but the marks that have to be aligned on the various scales are: one with a 5 on it and another without a number, between 2 and 3, to which you must assign the value. To act safely it is essential to have the help of an approximate mental operation. If you mentally calculate the squares of 1 and 2, you will be convinced that 2.25 is a reasonable value for the square of 1.5 and that therefore the operation has been done correctly. If, on the other hand, what is calculated is 4.2², it is evident that the answer cannot be 1.76, which is what the scale literally indicates, but that it must be greater than 10, and even 16, and therefore it is 17.6. You have to have the sense of the series of powers of 10; And if you don't have it, you have to buy it.
If the solution to the problem in which you are using the slide rule involves a series of chained operations, it is safest to write down the intermediate results on a piece of paper with a pencil. With some practice the cursor can also be used for these transfers in quite a few cases.
The exhaustive nature of nomographic solutions means that, if a nomogram can perform a certain arithmetic operation, it can also perform its inverse. Therefore, when one speaks of raising to powers one is simultaneously speaking of extracting roots of those same exponents, when of multiplication, also of division, etc. All that is required to go from one to the other is to apply the same procedure, changing the order of the scales.
Scales and typology
During the first two centuries of its existence, the slide rules were artisan products, manufactured individually and in very small quantities, if not unique. The functions for which they were prepared were therefore those requested by the inventor or the client (who often coincided in the same person) or those that the corresponding craftsman used to carry out. The first materials were noble woods that allowed the scales to be retained over time, mahogany was used in most cases. Some of the first rules were elaborated in engravings made in bone, and later brass was used.
As the 19th century progressed and scientific and technical knowledge and industrialization increased, the type of calculations performed by a growing number of civil and military engineers created a favorable market for the diffusion of this instrument. Thus, stable patterns emerged in the number and nature of the scales included in the rules that were offered commercially.
Types of Scales
The first of these was due to a French artilleryman, Amédée Mannheim, who designed the first truly popular slide rule in 1850. Part of this success was due to the inclusion in his model of the cursor, which was lacking in most of the preceding rules, which he did in 1851. This model was adopted by the French army and began to be manufactured industrially from 1859.
The scales are normally identified on the body of the ruler by an alphabetic symbol engraved on its left end. Without being absolutely uniform, this terminology is widely accepted by the various manufacturers and the following table details the most widespread designations and functions of the most common scales. In some cases the corresponding mathematical function is also specified, which is usually done at the right end of the scale.
The same is true for generic rule types. Although almost all models have additional scales, the three basic types and the scales they imply are:
- Mannheim: A, B, C, D.
- Rietz: A, B, C, D, K, L, S, T, ST, CI (path proposed by Max Rietz in 1902).
- Darmstadt: fundamentally adds LL scales to the Rietz model (a guide proposed by Alwin Walther in 1934).
Usual scales
| Designation | Description | Value |
| A | square scale; logarithmic scale of two dozens, located on the lower edge of the upper fixed strip | x2 |
| B | square scale, logarithmic scale of two dozens, located on the top edge of the mobile regette | x2 |
| C | duplicate of the base scale; logarithmic scale of a ten, located on the lower edge of the mobile regette | x |
| D | base scale; logarithmic scale of ten, located on the upper edge of the lower fixed strip | x |
| K | Cube scale; logarithmic scale of three dozen | x3 |
| CI | C scale "inverted", numbered from right to left; reciprocal scale | 1/x |
| CF | C scale "displaced"; its origin is a constant value different from the unit, usually pi or some submultiplo of its | (pi) * x |
| S | breast angles scale (in scale A) | sen−1x2 |
| T | Tangent angles scale (in scale A) | tg−1x2 |
| ST | scale of breasts and small angle tangents (0.58° to 5.73°); grade-radián conversions | arc x |
| L | linear scale used to obtain the common logarithms or decimal mantises (base 10) | log x |
| Ln | linear scale used for obtaining natural logarithms (base e) | ln x |
| LLn | set of double logarithmic scales (log-log), used for operations with exponents. They may have any basis (although it is usually number e) and are absolute (no need to estimate the decimal point position). | nx |
Specialized rules use many other scales, appropriate to the calculations for which they are intended (eg statistical or electrical engineering), sometimes ignoring some of the above.
| ||
| Scales of the anterior and posterior faces of a duplex rule K strangerE 4081-3. |
Theoretical foundation
It is convenient to group into two different categories the mathematical operations that can be carried out with the slide rule.
Static nomograms
In one of them the scales work like those of a simple nomogram, remaining fixed, and the only thing that has to be moved to obtain the results is the cursor or the string, although in many cases they could also be achieved without it. This is what happens, for example, in a ruler that has the scale D on the lower ruler and A on the upper one (that is, practically anywhere).. The operations of squaring and obtaining the square root of a number do not require the mobile ruler at all and sometimes not even the cursor, and many of them can be done by eye. These operations are represented by equations of two variables, y = f(x), where the function can be the exponentiation, any of the trigonometric, obtaining logarithms, etc.
Sliding scales
Operations in the second category require movements of the intermediate slider. D'Ocagne called nomo-mechanical instruments those that use some simple mechanical resource to produce the geometric coincidences required by the use of a nomogram. This is what happens in the present case.
Such operations are reduced to two, namely addition and multiplication (with their inverses subtraction and division). This additional requirement derives from the fact that they are the only equations of three variables, z = f(x, y)), which are usually solved with the slide rule. In these cases what is substantially done is an addition (or subtraction) of linear segments.
Segment addition
It is often said that the slide rule is not useful for adding, which is true to some extent, but it does not contradict the preceding statement. To be convinced of this, it is enough to slide the scales of two ordinary rulers one over the other. If, for example, the initial mark of the upper scale is located in front of the number 3 of the lower one, it will be verified that now each of the other numbers of the upper scale is in front of another one of the lower one that is equal to the sum of himself and 3.
If the ordinary slide rule does not have scales to carry out additions, it is not because of an inherent incapacity, but for the following two reasons: 1) addition is an operation that everyone is educated to perform mentally, and almost unconscious mode, when it only has two factors and these are not excessively large; and 2) if addition scales were included in the slide rule, the length of the addition would have to be very inconvenient for the fact to be of any use. For example, a slide rule 25 cm long could not perform sums greater than "16+9" ("160+90" if you used the mm marks), for which no one usually needs any mechanical help. (To counteract this limitation, the European manufacturer Faber-Castell attached to the back of some of its models a simple six-digit mechanical and digital adding machine, called the Addiator, during a period between 1950 and 1970 approximately).
Multiplication
What needs to be explained is rather the opposite. That is, if what the sliding slide rule primarily does is addition, how can it be used to multiply and divide? It seems that there must be some hidden trick. And indeed there is; it is a nomographic trick, which consists of calibrating the scales used in another way. To understand it well, let's follow this calibration process step by step, for which it is convenient to return to the usual scale of an ordinary ruler, graduated in cm.
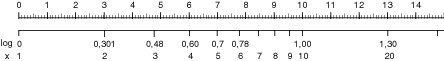
Let's place another line under it, which we will mark according to the values of the decimal logarithms of the numbers of the first scale. This second scale has its origin, its point 0, coinciding with that of the first, placing its remaining marks at the height that corresponds to them in the first according to the values of the successive logarithms, some of which appear written in the following line, labeled "log", to the left of the lower figure. And for greater clarity, the corresponding figure can still be placed below, a line that is labeled as "x" in this figure. In short, it is a graphical representation of the equation y = log x. The first line of figures represents the values of y and the second those of x.
Since what is of interest here is not the absolute value of the digits of y, but the relative spacing of the values, the picture can be cleared up by suppressing the first indication and leaving only the values of the first indication visible. independent variable, x, like this:
This was what Edmund Gunter did in 1620, inscribing a similar one in a rule-shaped mathematical instrument, more than half a meter long, which also contained various other scales useful for mercantile and marine practice. The calculations were carried out by applying the magnitudes of the factors to them by means of compasses, an almost unimaginable way of proceeding today, but which was very common at the time.
If the segment addition process is repeated now using two of these scales, the result is very different from what was obtained before:
The upper scale has moved 1.5 units above the lower one, but the figure obtained under each upper mark is now not the corresponding number added to 1.5, but the of said number multiplied by 1.5. The miracle is done. Probably more appropriate to say the magic, which consists of two parts. On the one hand, the logarithmic calibration transmutes the sum of segments into multiplication, by the property of logarithms that is formulated: log a + log b = log (a x b). On the other hand, the labeling at the end of the scale gives it the appearance that it refers directly to the numbers; the logarithms disappear from the scene and everything returns to its initial arithmetic aspect.
Contenido relacionado
Chimu mythology
Soufriere district
Classical economics