Riemann zeta function

Riemann zeta function φ(s) in the complex plane. The color of a point s encodes the value of ius(s). Strong colors denote values close to 0 and the tone encodes the value of the argument. The white point in s=1 is the pole of the zeta function; black points in the negative real axis and in the critical line Re(s) = 1/2 are their zeros.

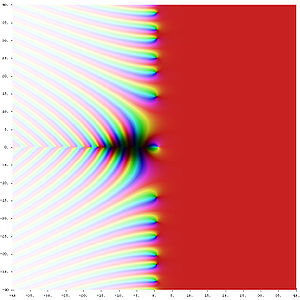
The Riemann zeta function (often referred to as dseta for transliteration of the Greek letter ζ), named after Bernhard Riemann, is a function of important significant in number theory, because of its relationship with the distribution of prime numbers. It also has applications in other areas such as physics, probability theory, and applied statistics.

Definition

The Riemann zeta function ζ(s) is defined, for complex values with real part greater than one, by the Dirichlet series:

γ γ (s)=␡ ␡ n=1∞ ∞ 1ns{displaystyle zeta (s)=sum _{n=1}^{infty}{frac {1}{n^{s}}}}}}

In the region {s ∈ C | Re(s) > 1}, this infinite series converges and defines a function that is analytic in this region. Riemann observed that the zeta function can be uniquely extended by analytic continuation to a meromorphic function on the entire complex plane with a single pole at s = 1. This is the function considered in the hypothesis of Riemann.

For complexes with Re(s)<1, the values of the function must be calculated by means of its functional equation, obtained from the analytic continuation of the function.

Relationship with prime numbers

The connection between this function and prime numbers was first observed by Leonhard Euler, who realized that:

11s+12s+13s+...... =␡ ␡ n≥ ≥ 11ns= p␡ ␡ k≥ ≥ 0(p− − s)k=(1+12s+14s+ )(1+13s+19s+ ) (1+1ps+1p2s+1p3s+ ) {cHFFFFFF}{1⁄2⁄2}{cHFFFFFF}{cHFFFFFF}{cHFFFFFF}{cHFFFFFF00}{cHFFFFFF00}{cHFFFFFFFF00}{cHFFFFFF00}{cHFFFFFFFFFFFFFF}{cHFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFF}{c}{c}{c}{c}{cH00}{cHFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFF00}{c}{c}{c}{c}{cH00}{cHFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFF00}{c}{c}{c}{cHFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFF

Since for every cousin p, ␡ ␡ k≥ ≥ 0(p− − s)k{displaystyle textstyle sum _{kgeq 0}(p^{-s})^{k}}} is a geometric series, convergent for any complex number s with Re(s)  1 to:

␡ ␡ k≥ ≥ 0(p− − s)k=11− − p− − s{displaystyle sum _{kgeq}(p^{-s})^{k}={frac {1}{1-p^{-s}}}}}}

it is obtained that:

␡ ␡ n≥ ≥ 11ns= p한 한 P11− − p− − s{displaystyle sum _{ngeq 1}{frac {1}{n^{s}=}{pin mathbb {P}{frac {1}{1}{1-p^{-s}}}}}}}}}

where the infinite product is over all prime numbers and s is a complex number with Re(s) > 1. This expression is called the Euler product, after its discoverer. The formula is a consequence of two simple but fundamental results in Mathematics: the formula for geometric series and the fundamental theorem of arithmetic.

Basic properties

Riemann zeta function s  1 with s real

Some values

Euler was able to find a closed formula for ζ(2k) when k is a positive integer:

γ γ (2k)=(− − 1)k− − 1(2π π )2kB2k2(2k)!{displaystyle zeta (2k)={frac {(-1)^{k-1}(2pi)^{2}B_{2k}}{2(2,k)}}}}}}}}{2(2,k)}}}}

where B_2k are the Bernoulli numbers. From this formula it is obtained that: ζ(2) = π²/6, ξ(4) = π⁴/90, ξ(6) = π⁶/945 etc.. For odd numbers there is no known general solution.

For negative values, if k ≥ 1, then

γ γ (− − k)=− − Bk+1k+1{displaystyle zeta (-k)=-{frac {B_{k+1}}{k+1}}}}}

It can be seen that for negative even numbers, the Riemann zeta function vanishes, these being called trivial zeros.

γ γ (− − 1)=− − 1/12{displaystyle zeta (-1)=-1/12!}

γ γ (1)=1+12+13+ =∞ ∞ {displaystyle zeta (1)=1+{frac {1}{2}}} +{frac {1}{3}{3}}+cdots =infty !} corresponds to the harmonic series.

γ γ (3)=1+123+133+ ≈ ≈ 1.202;{displaystyle zeta (3)=1+{frac {1}{2^{3}}}}{3^{3}}}}}+cdots approx 1.202;!} It's Apéry's constant.

Functional Equation

The Riemann zeta function can be extended analytically for all complex numbers except s=1, by the following functional equation:

γ γ (s)=2sπ π s− − 1without (π π s2)Interpreter Interpreter (1− − s)γ γ (1− − s){displaystyle zeta (s)=2^{s}pi ^{s-1}sin left({frac {pi s}{2}}}right)Gamma (1-s)zeta (1-s)!}

The equation has a simple pole s=1 with residue 1 and proved by Bernhard Riemann in 1859 in his essay About the number of minor prime numbers than a given amount. An equivalent relationship was conjectured by Euler for the function ␡ ␡ n=1∞ ∞ (− − 1)n+1ns{displaystyle textstyle sum _{n=1}^{infty }{frac {(-1)^{n+1}}{n^{s}}}}}}}}.

There is also a symmetrical version of the functional equation under change s (1− − s){displaystyle textstyle smapsto (1-s)}.

γ γ (s)Interpreter Interpreter (s2)π π − − s2=γ γ (1− − s)Interpreter Interpreter (1− − s2)π π − − 1− − s2{displaystyle zeta (s)Gamma left({frac {s}{2}right)pi ^{-{frac {s}{2}}}}=zeta (1-s)Gamma left({frac {1-s}{2}}{2}}{2}{right)pi ^{-{frac {1-s}{1}{1}{1}}}{1}}}{1}{frac}}}}}}}{

where Γ(s) is the gamma function.

Sometimes the function is defined:

roga roga (s)=π π − − s/2Interpreter Interpreter (s2)γ γ (s){displaystyle xi (s)=pi ^{-s/2}Gamma left({frac {s}{2}}right)zeta (s)!}

with what

roga roga (s)=roga roga (1− − s){displaystyle xi (s)=xi (1-s)!}

The functional equation also satisfies the following asymptotic limit:

γ γ (1− − s)=(s2π π e)s8π π s# (π π s2)(1+O(1s)){displaystyle zeta left({1-s}right)=left({frac {s}{2pi e}}}{s}{sqrt {frac {8pi }{s}{s}}{cHFFFFFF}{cHFFFF}{cHFFFFFF}{1}{cHFFFFFFFFFFFFFFFFFFFFFF}{c}}{c}{cHFF}{cHFFFFFFFFFFFFFFFFFF}{cHFFFFFFFFFFFFFFFFFFFFFFFFFFFF}{cHFF}{cHFF}{c}{}{cHFFFFFFFFFFFFFFFFFFFFFFFF}{cH00}{cHFFFFFF}{cHFFFFFFFFFFFFFF}{}{cHFF}{c}{}{}{cH00}{cHFFFFFFFF}{cHFF

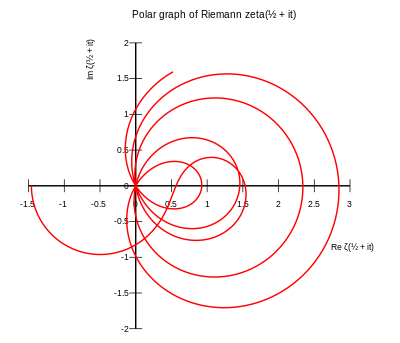
Function zeros

This image shows a polar chart of Riemann's zeta function along the critical straight for values t between 0 and 34. The first five zeros are clearly visible, since they correspond to the passage of the spiral by the origin.

The value of the zeta function for negative even numbers is 0 (seeing the functional equation is self-evident), so they are called trivial zeros. Apart from trivial zeros, the function also aborts on values of s that are within the range {s ∈ C: 0 < Re(s) < 1}, and which are called nontrivial zeros, since it is more difficult to prove the location of those zeros within the critical range. The study of the distribution of these "non-trivial zeros" is very important, because it has profound implications for the distribution of prime numbers and for questions related to number theory. The Riemann hypothesis, considered one of the largest mathematical problems currently open, ensures that any non-trivial zero has to fulfill Re(s)=1/2, therefore, all the zeros are aligned in the complex plane forming a line, called critical line.

The location of these zeros is of significant importance in number theory, since, for example, the fact that all zeros are in the critical range proves the prime number theorem. A better result is that ζ(σ + it) ≠ 0 for any |t| ≥ 3 and

σ σ ≥ ≥ 1− − 157.54(log 日本語t日本語)2/3(log log 日本語t日本語)1/3{displaystyle sigma geq 1-{frac {1}{57.54(log {ATA})^{2/3}(log {log {excHFFFF}{1}}}{1/3}}}}{!}

It is also known that there are infinitely many zeros on the critical line, as G.H. Hardy and Littlewood.

A method for Convergent Series with values less than unity y (s = 1) is already known by Andri Lopez.

γ γ (s)=(12)2+[chuckles]i(13+16)]2=0{displaystyle zeta(s)=({frac {1}{2}}}})^{2}+[i({frac {1}{3}}}{frac {1}{1}{6}}}})]{2}=0}}

The series starts with these two addends and we have the first absolute zero. For the extension of the series, the progressive substitution method is applied: substituting the last fraction for two fractions that have said fraction as root (that is, substituting the value of 1/6).

Reciprocal of the function

The reciprocal of the zeta function can be expressed by a Dirichlet series over the Möbius function μ(n) defined for any complex number s with the real part greater than 1 like:

1γ γ (s)=␡ ␡ n=1∞ ∞ μ μ (n)ns{displaystyle {frac {1}{zeta(s)}}=sum _{n=1^}{infty }{frac {mu (n)}{n^{s}}}}}}}}}}{

There are other expressions of this type that make use of multiplicative functions, such as

γ γ (s− − 1)γ γ (s)=␡ ␡ n=1∞ ∞ φ φ (n)ns{displaystyle {frac {zeta(s-1)}{zeta(s)}}}=sum _{n=1^{infty }{frac {varphi(n)}{n^{s}}}}}}}}}{

where φ(n) is the Euler function φ.

Universality

The zeta function has the remarkable property of universality. This universality says that there exists some location within the critical range that approximates any holomorphic function quite well. Since this type of function is quite general, this property is quite important.

Representations

The Riemann zeta function has different representations, some of which are shown below:

Mellin transform

• For values s with the larger real part you have to

γ γ (s)=1Interpreter Interpreter (s)∫ ∫ 0∞ ∞ (xs− − 1Exp (x)− − 1)dx{displaystyle zeta (s)={frac {1}{Gamma (s)}int _{0}^{infty }left({frac {x^{s-1}}{exp(x)-1}{right) dx!}

The Mellin Transformation of Function 1/(exp(x)-1) is precisely the previous expression. I mean:

{M(1Exp (x)− − 1)!(s)=Interpreter Interpreter (s)γ γ (s){displaystyle left{mathcal {M}}left({frac {1}{exp(x)-1}}}}rightright}(s)=Gamma (s)zeta (s)zeta!}

• It can also relate to the prime numbers and the theorem of the prime numbers. If π(x) is the counting function of prime numbers, then:

log γ γ (s)=s∫ ∫ 0∞ ∞ π π (x)x(xs− − 1)dx{displaystyle log zeta (s)=sint _{0}^{infty }{frac {pi (x)}{x(x^{s}-1)}}{,dx}

convergent for Re() valuess) If ω() function is definedsLike

ω ω (s)=∫ ∫ 0∞ ∞ π π (x)xs+1(xs− − 1)dx{displaystyle omega (s)=int _{0}^{infty }{frac {pi(x)}{x^{s+1}(x^{s}-1)}}}}{ dx!}

then the transformation of Mellin

{Mπ π (x)!(− − s)=log γ γ (s)s− − ω ω (s).{displaystyle left{mathcal {M}}pi (x)right}(-s)={frac {log zeta (s)}{s}-omega (s). !

• A similar Mellin transformation, which relates Riemann's cousin counting function, defined as J(x)=␡ ␡ π π (x1/n)n.{displaystyle textstyle J(x)=sum {frac {pi (x^{1/n}}{n}. ! is:

{MJ(x)!(− − s)=log γ γ (s)s{displaystyle left{mathcal {M}}J(x)right}(-s)={frac {log zeta (s)}{s}}!}

Laurent Series

The zeta function is meromorphic with a simple pole at s=1. This can be expanded as a Laurent series around s=1, the resulting series is:

γ γ (s)=1s− − 1+␡ ␡ n=0∞ ∞ (− − 1)nn!γ γ n(s− − 1)n{displaystyle zeta(s)={frac {1}{s-1}}}}+sum _{n=0}^{infty }{frac {(-1)^{n}}{n}}}}{n}}}{n}{n}{n}{n}{n}{n}}{n}}}{n}{n}}{n}{n}{n}}}}{

where the constants γ_n, are called Stieltjes constants and are defined as:

γ γ n=limm→ → ∞ ∞ ((␡ ␡ k=1m(log k)nk)− − (log m)n+1n+1){displaystyle gamma _{n}=lim _{mrightarrow infty}{left(left(sum _{k=1}^{m}{frac {(log k)^{n}}{k}}{k}}{right)}-{frac {(log m){n+1}{n+1}}}}}}}}{nright}}}}}}}}}

The constant γ₀ corresponds to the Euler-Mascheroni constant.

Product of Hadamard

Using the Weierstrass factorization theorem, Hadamard gave an infinite product expansion of the zeta function:

γ γ (s)=e(log (2π π )− − 1− − γ γ /2)s2(s− − 1)Interpreter Interpreter (1+s/2) ρ ρ (1− − sρ ρ )es/ρ ρ ,{displaystyle zeta (s)={frac {e^{(log(2pi)-1-gamma /2)s}{2(s-1)Gamma (1+s/2)}}}{prod _{rho }{left(1-{frac {s}{rho }}{right)e^{s/rho },

where the product is over all non-trivial zeros ρ of ζ and the letter γ corresponds to the Euler-Mascheroni constant. A simpler way is:

γ γ (s)=π π s/2 ρ ρ (1− − sρ ρ )2(s− − 1)Interpreter Interpreter (1+s/2){displaystyle zeta (s)=pi ^{s/2}{frac {prod _{rho}left(1-{frac {s}{rho }}}{right)}{2(s-1)Gamma (1+s/2)}}}{!}

In this elegant way we can observe the simple pole at s=1 (denominator), the trivial zeros given by the term of the gamma function (denominator), and the non-trivial zeros, given when s=ρ (numerator).

Global String

A series representation, convergent for all complex number s, except 1, was conjectured by Konrad Knopp and proved by Helmut Hasse in 1930:

γ γ (s)=11− − 21− − s␡ ␡ n=0∞ ∞ 12n+1␡ ␡ k=0n(− − 1)k(nk)(k+1)− − s.{displaystyle zeta (s)={frac {1}{1-2^{1-s}sum _{n=0}^{infty }{frac {1}{2^{n+1}}}}{k=0}{n}{n}{k}{n choose k(k+1)^{-s}}{. !

Applications

Although mathematicians consider the zeta function to be of primary interest in the "purest" of mathematical disciplines, number theory, it also has applications in statistics and physics. In some calculations performed in physics, the sum of positive integers must be evaluated. Paradoxically, for physical reasons a finite response is expected. When this situation occurs, there is usually a rigorous approach with in-depth analysis, as well as a "shortcut", using the Riemann zeta function. The argument is the following:

• We want to evaluate the sum 1 + 2 + 3 + 4 +... but we can rewrite it as a sum of its reverses.

S=1+2+3+4+ =(11)− − 1+(12)− − 1+(13)− − 1+(14)− − 1+ =␡ ␡ n=1∞ ∞ 1n− − 1{displaystyle S=1+2+3+4+cdots =left({frac {1}{1}{1}{1}{1} +left({frac {1}{1}{2}{1}{1}{1⁄2}{1}{1}{1⁄2}{1⁄2}{1⁄2}}{1⁄2}{1⁄2}}{1⁄2}}}}{1⁄2}{1⁄2}}}}{1⁄2}}{1⁄2}}}{1⁄2}}}{1⁄2}}}}{1⁄2}}{1⁄2}}{1⁄2}}{1⁄2}}{1⁄2}}{1⁄2}}}}}}}}}}{1⁄2}}{1⁄2}{1⁄1⁄2}}}}{1⁄2}{1⁄1⁄2}}}}}{1⁄2}}}{1⁄1⁄2}}}}}{

The amount S It seems to take the form γ γ (− − 1){displaystyle textstyle zeta (-1)}. However, −1 leaves out of the convergence domain of the Dirichlet series for the zeta function. However, a divergent series with positive terms like this can sometimes to be represented reasonably by the Ramanujan summation method. This method of sum involves the application of the Euler-Maclaurin formula, and when applied to the zeta function, its definition extends to the entire complex plane. In particular,

1+2+3+ =− − 112(R R ){displaystyle 1+2+3+cdots =-{frac {1}{12}(Re)}

where the notation (R R ){displaystyle textstyle (Re)} indica sum of Ramanujan. For pair exponents you have to:

1+22k+32k+ =0(R R ){displaystyle 1+2^{2k}+3^{2k}+cdots =0(Re)}!

and for odd exponents, the relationship with the Bernoulli numbers is obtained:

1+22k− − 1+32k− − 1+ =− − B2k2k(R R ).{displaystyle 1+2^{2k-1}+3^{2k-1}+cdots =-{frac {B_{2k}}{2k}}(Re). !

Zeta function regularization is used as a possible means of regularizing divergent series in quantum field theory. As a notable example, the Riemann zeta function appears explicitly in the calculation of the Casimir effect.

Generalizations

There are a number of zeta functions that can be related to the Riemann zeta function. These include:

• The Hurwitz zeta function, defined as:

γ γ (s,q)=␡ ␡ k=0∞ ∞ (k+q)− − s{displaystyle zeta (s,q)=sum _{k=0}^{infty }(k+q)^{-s}!}

that matches Riemann's zeta function when q=1 (Note that the lower limit of the sum is 0 and not 1).

• The polylogaritme, which is given by

Lis(z)=␡ ␡ k=1∞ ∞ zkks{displaystyle mathrm {Li} _{s}(z)=sum _{k=1^{infty}{z^{k} over k^{s}}!}

and that corresponds to the Riemann zeta function when z=1.

• Lerch's zeta function, given by:

≈ ≈ (z,s,q)=␡ ␡ k=0∞ ∞ zk(k+q)s{displaystyle Phi (z,s,q)=sum _{k=0}^{infty }{frac {z^{k}{(k+q)^{s}}}}}}}}{!}

and that corresponds to the Riemann zeta function when q=1 and z=1.

Among the generalizations we can find the Dirichlet L functions or the Dedekind zeta function, as well as multiple zeta functions, defined as:

k_{2}>cdots >k_{n}>0}k_{1}^{-s_{1}}k_{2}^{-s_{2}}cdots k_{n}^{-s_{n}}!}" xmlns="http://www.w3.org/1998/Math/MathML">γ γ (s1,s2,...... ,sn)=␡ ␡ k1▪k2▪ ▪kn▪0k1− − s1k2− − s2 kn− − sn{displaystyle zeta (s_{1},s_{2},dotss_{n})=sum _{k_{1}{2}{2}{2}{2}{2}{cdots 한k_{n}{n1}{1^{1}{1}{2^}{-s_{2}{2}{cdots k_{n}{c}{n}{cdots}{1⁄2}{n}{1}{1⁄2}{?k_{2}>cdots >k_{n}>0}k_{1}^{-s_{1}}k_{2}^{-s_{2}}cdots k_{n}^{-s_{n}}!}" aria-hidden="true" class="mwe-math-fallback-image-inline" src="https://wikimedia.org/api/rest_v1/media/math/render/svg/ae53d07d99f85c23b17803d52d3d9b4943e934b4" style="vertical-align: -3.338ex; margin-right: -0.387ex; width:48.864ex; height:5.843ex;"/>

One can analytically extend these functions to a complex n-dimensional space. The special values of these functions have been used to connect different branches of mathematics and physics.