Geometry
The geometry (from the Latin geometrĭa, and this from the Greek γεωμετρία from γῆ gē, 'earth', and μετρία metry, 'measurement') is a branch of mathematics that deals with the study of the properties of figures in the plane or space, including: points, lines, planes, polytopes (such as parallels, perpendiculars, curves, surfaces, polygons, polyhedra, etc.).
It is the theoretical basis of descriptive geometry or technical drawing. It also provides the basis for instruments such as the compass, theodolite, the pantograph or the global positioning system (especially when considered in combination with mathematical analysis and especially with differential equations).
Its origins go back to the solution of specific problems related to measurements. It has its practical application in applied physics, mechanics, architecture, geography, cartography, astronomy, nautical, topography, ballistics, etc., and is useful in preparing designs and even in making handicrafts.
History
Babylonian civilization was one of the first cultures to incorporate the study of geometry. The invention of the wheel opened the way to the study of the circumference and later to the discovery of the number π (pi); They also developed the sexagesimal system, knowing that each year has 365 days, also implemented a formula to calculate the area of the rectangle trapeze.
In ancient Egypt it was very developed, according to the texts of Herodotus, Strabon and Diodore. Euclides, in the centuryIIIa. C. configured geometry in axiomatic and constructive form, a treatment that established a rule to follow for many centuries: euclidian geometry described in The Elements.
The study of astronomy and cartography, trying to determine the positions of stars and planets in the celestial sphere, served as an important source of solving geometric problems for more than a millennium. Rene Descartes simultaneously developed the algebra of equations and analytical geometry, marking a new stage, where geometric figures, such as flat curves, could be represented analytically, that is, with functions and equations. Geometry is enriched by the study of the intrinsic structure of the geometric ents analyzed by Euler and Gauss, which led to the creation of topology and differential geometry.Axioms, definitions and theorems
Geometry aims to go beyond what is achieved by intuition. For this reason, a rigorous method is necessary, without errors; to achieve this, axiomatic systems have been used historically. The first axiomatic system is established by Euclid, although it was incomplete. David Hilbert proposed at the beginning of the XX century another axiomatic system, this one now complete. As in any formal system, definitions are not only intended to describe the properties of objects, or their relationships. When something is axiomatized, objects become ideal abstract entities and their relationships are called models.
This means that the words «point», «line» and «plane» must lose all material meaning. Any set of objects that verifies the definitions and axioms will also satisfy all the theorems of the geometry in question, and their relationships will be virtually identical to that of the "traditional" model.
The following are some of the most important concepts in geometry.
Axioms
In Euclidean geometry, axioms and postulates are propositions that relate concepts, defined in terms of the point, the line, and the plane. Euclid raised five postulates and it was the fifth (the parallelism postulate) that centuries later —when many geometers questioned it when analyzing it— gave rise to new geometries: the elliptic (Riemann's geometry) or the hyperbolic of Nikolai Lobachevski.
In analytic geometry, axioms are defined in terms of equations of points, based on mathematical analysis and algebra. It acquires another new meaning to talk about points, lines or planes. f(x) can define any function, call it a line, circle, plane, etc.
Euclid took an abstract approach to geometry in his Elements, one of the most influential books ever written. Euclid introduced certain axioms or postulates that express primary or self-evident properties of points, lines, and planes. He proceeded to rigorously deduce other properties by mathematical reasoning. The characteristic feature of Euclid's approach to geometry was its rigor, and it has come to be known as axiomatic or synthetic geometry. In the early 19th century, the discovery of non-Euclidean geometries by Nikolai Ivanovich Lobachevsky (1792-1856), János Bolyai (1802-1860), Carl Friedrich Gauss (1777-1855) and others led to a revival of interest in the discipline, and in the century XX, David Hilbert (1862 –1943) used axiomatic reasoning in an attempt to provide a modern basis for geometry.
Points
Points are considered fundamental objects in Euclidean geometry. They have been variously defined, including Euclid's definition as "that which has no part" and by using algebra or nested sets. In many areas of geometry, such as analytic geometry, differential geometry, and topology, all objects are considered to be constructed from points. However, some study of geometry without reference to points has been made.
Lines
Euclid described a line as "length without width" that "is found equally with respect to the points on itself". In modern mathematics, given the multitude of geometries, the concept of a line is closely related to the way geometry is described. For example, in analytic geometry, a line in the plane is often defined as the set of points whose coordinates satisfy a given linear equation, but in a more abstract setting, such as incidence geometry, a line can be an independent object., distinct from the set of points that lie on it. In differential geometry, a geodesic is a generalization of the notion of line to curved spaces.
Plans
A plane is a two-dimensional flat surface that extends infinitely. Planes are used in all areas of geometry. For example, planes can be studied as a topological surface without reference to distances or angles; It can be studied as an affine space where collinearity and proportions can be studied but not distances; It can be studied as the complex plane using complex analysis techniques; and so on.
Angles
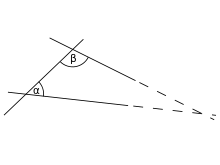
Euclid defines a plane angle as the inclination to each other, in a plane, of two lines that meet and are not straight to each other. In modern terms, an angle is the figure formed by two rays of light, called sides of the angle, that share a common endpoint, called the vertex of the angle.
In Euclidean geometry, angles are used to study polygons and triangles, as well as forming an object of study in their own right. The study of angles in a triangle or angles in a unit circle forms the basis of trigonometry.
In differential geometry and calculus, angles between plane curves or space curves or surfaces can be calculated using the derivative
Curves
A curve is a one-dimensional object that can be straight (like a line) or not; curves in two-dimensional space are called plane curves, and those in three-dimensional space are called space curves.
In topology, a curve is defined by a function from a range of real numbers to another space. In differential geometry, the same definition is used, but the defining function is required to be differentiable. Algebraic geometry studies algebraic curves, which are defined as algebraic manifolds of dimension one.
Surfaces
A surface is a two-dimensional object, such as a sphere or a paraboloid. In differential geometry and topology, surfaces are described by "patches" two-dimensional (or neighborhoods) that are assembled by diffeomorphisms or homeomorphisms, respectively. In algebraic geometry, surfaces are described by polynomial equations.
Varieties
A manifold is a generalization of the concepts of curve and surface. In topology, a manifold is a topological space where every point has a neighborhood that is homeomorphic to the Euclidean space. In differential geometry, a differentiable manifold is a space where each neighborhood is diffeomorphic to the Euclidean space.
Manifolds are widely used in physics, including general relativity and string theory.
Length, Area, and Volume
Length, area, and volume describe the size or extent of an object in one dimension, two dimensions, and three dimensions, respectively.
In Euclidean geometry and analytic geometry, the length of a line segment can often be calculated using the Pythagorean theorem.
Area and volume can be defined as fundamental quantities separate from length, or they can be described and calculated in terms of lengths in a plane or three-dimensional space. Mathematicians have found many explicit formulas for the area and formulas for the volume of various geometric objects. In calculus, area and volume can be defined in terms of integrals, such as the Riemann integral or the Lebesgue integral.
Metrics and measurements
The concept of length or distance can be generalized, giving rise to the idea of metrics. For example, the Euclidean metric measures the distance between points in the Euclidean plane, while the hyperbolic metric measures the distance in the hyperbolic plane. Other important examples of metrics include the Lorentz metric of special relativity and the Semi-Riemannian metric of general relativity.
In another direction, the concepts of length, area, and volume are expanded with measure theory, which studies methods of assigning a size or measure to sets, where the measures follow rules similar to those of classical area and volume.
Congruence and similarity
Congruence and similarity are concepts that describe when two shapes have similar characteristics. In Euclidean geometry, similarity is used to describe objects that have the same shape, while congruence is used to describe objects that are the same in both size and shape. Hilbert, in his work on creating a more rigorous basis for geometry, treated congruence as an undefined term whose properties are defined by axioms.
Congruence and similarity are generalized to transformation geometry, which studies the properties of geometric objects that are preserved by different types of transformations.
Compass and ruler constructions
Classical geometers paid special attention to the construction of geometric objects that had been described in some other way. Classically, the only instruments allowed in geometric constructions are the compass and the ruler. Furthermore, each build had to be completed in a finite number of steps. However, some problems proved difficult or impossible to solve by these means alone, and ingenious constructions were found using parabolas and other curves, as well as mechanical devices.
Dimension
Where traditional geometry allowed dimensions 1 (a line), 2 (a plane), and 3 (our ambient world conceived as three-dimensional space), higher dimensions have been used by mathematicians and physicists for nearly two centuries. An example of mathematical use for higher dimensions is the configuration space of a physical system, which has a dimension equal to the degrees of freedom of the system. For example, the configuration of a screw can be described by five coordinates.
In general topology, the concept of dimension has been extended from the natural numbers to the infinite dimension (Hilbert spaces, for example) and the positive real numbers (in fractal geometry). ]]). In algebraic geometry, the dimension of an algebraic manifold has been given several apparently different definitions, all of which are equivalent in the most common cases.
Symmetry
The subject of symmetry in geometry is almost as old as the science of geometry itself. Symmetrical shapes such as the circle, regular polygons, and the Platonic solids had deep meaning for many ancient philosophers and were investigated in detail before the time of Euclid. Symmetrical patterns occur in nature and were artistically represented in a multitude of forms, including the graphics of Leonardo da Vinci, MC Escher, and others. In the second half of the century XIX, the relationship between symmetry and geometry was the subject of intense scrutiny.
Felix Klein's Erlangen program proclaimed that, in a very precise sense, symmetry, expressed through the notion of a transformation group, determines what geometry is. Symmetry in classical Euclidean geometry is represented by congruences and rigid motions, while in projective geometry an analogous role is played by collinations, geometric transformations that turn straight lines into straight lines. However, it was in the new geometries of Bolyai and Lobachevsky, Riemann, Clifford, and Klein, and Sophus Lieque Klein's idea of "defining a geometry through its symmetry group" he found inspiration from him.Both discrete and continuous symmetries play a prominent role in geometry, the former in topology and geometric group theory, the latter in Lie theory and Riemannian geometry.
A different type of symmetry is the principle of duality in projective geometry, among other fields. This meta-phenomenon can be roughly described as follows: in any theorem, swap ”point” with “flat”, “join” with “meet”, “meets” with “contains”, and the result is an equally theorem. true. A similar and closely related form of duality exists between a vector space and its dual space.
Topology and geometry
The field of topology, which developed greatly in the 20th century, is in a technical sense a kind of transformational geometry, in which transformations that preserve the properties of figures are homeomorphisms (for example, this differs from metric geometry, in that transformations that do not alter the properties of figures are isometries). This has often been expressed in the form of the saying: "topology is the geometry of the rubber page".
Types of geometry
Since the ancient Greeks, there have been numerous contributions to geometry, particularly from the 18th century. This has caused numerous sub-branches of geometry to proliferate with very different approaches. Different approaches can be used to classify the different developments of modern geometry:
Geometries according to the type of space
The ancient Greeks handled only one type of geometry, namely Euclidean geometry, cleverly codified in Euclid's Elements by an Alexandrian school headed by Euclid. This type of geometry was based on a formal style of deductions from five basic postulates. The first four were widely accepted and Euclid used them extensively, however, the fifth postulate was less used and later various authors tried to demonstrate it from the others, the impossibility of said deduction led to the verification that along with Euclidean geometry there were other types of geometries in which Euclid's fifth postulate did not participate. According to the modifications introduced in this fifth postulate, different families of geometries or different geometric spaces between them are reached:
- Absolute geometry, which is the set of geometric derivative facts from only the first four postulates of Euclides.
- Euclid geometry, which is the particular geometry that is obtained from accepting as axiom also the fifth postulate. The Greeks considered two variants of Euclidean geometry:
- Euclid geometry of the plane
- Euclid geometry of space
- Classical geometry is a collection of results for Euclidean geometries.
From the 19th century it was concluded that non-Euclidean geometries could be defined among themselves:
- Elliptical geometry
- The spherical geometry
- The finite geometry
- Hyperbolic geometry
- The riemannian geometry
Geometries associated with transformations
In the 19th century, Klein developed the so-called Erlange Program that established another way of approaching geometric concepts: studying under which different types of mathematical transformations invariances were verified. Thus, groups endowed with various operations were identified and subdisciplines were raised based on which were the particular types of transformations under which invariances were recorded. This study allowed the following geometric classification:
- Affin geometry
- Consistent geometry
- Convex geometry
- Discreet geometry
- Incidence geometry
- Ordained geometry
- Projective geometry
Geometry according to the type of representation
Although Euclid was basically restricted to geometric concepts that could be represented by figures (points, lines, circles, etc.) the development of other branches of mathematics not initially connected with geometry itself, led to the ability to apply the tools of other Branches to properly geometric problems were thus born: . algebraic geometry . analytical geometry . descriptive geometry
- The geometric topology
- The differential geometry that encompasses as branches to:
- Discreet differential geometry
- The geometry of curves and surfaces
- The differential geometry of curves
- Differential Geometry of Surfaces
- The differential geometry of hypersurfaces
- Differential geometry of varieties
- The geometry of Riemann
- Fractal Geometry
- Synthetic geometry
Geometric applications
In addition to the subbranches themselves, numerous practical applications of geometry have arisen in modern times, among them:
- Computational geometry
- Constructive geometry of solids
- Molecular geometry
Teaching and learning geometry
Learning geometry involves developing visual and reasoning skills.
In order for the learning of geometry not to be meaningless, it is important that the teaching group is concerned with finding a balance between the association of visualization and argumentation skills, since both skills are fundamental within the individual's training process. In other words, it is not just about teaching content as a "recipe" or to comply with what is stipulated in the curriculum, but it is intended that with the teaching of geometry the student body learns to think logically.
Human beings, from childhood, create representations of the physical world around them. These generate a need (theoretical and practical) to achieve an understanding of that world. The right hemisphere of the brain turns out to be the most benefited in the presence of visual stimuli, unlike the left hemisphere, which is responsible for developing verbal abilities. The study of geometry contributes significantly to the development of those visualization spatial needs; however, until a recent historical period, dating from the 1950s, is when mathematics educators became interested in the study of this field, linking mathematical ability with spatial ability.
Regarding the difficulties that students present when studying geometry are: solving a problem algebraically; calculate perimeters, areas and volumes, because they do not identify which formula to apply and difficulty in interpreting what a problem says. When carrying out the analysis by level, it can be observed that in the diversified cycle (tenth and eleventh year) the main difficulty they present is interpreting what a problem says. The main difficulty of the students of seventh, eighth and ninth year, is, respectively, to understand the formulas of the perimeter, areas and volumes and to learn the definitions; solve a problem situation algebraically and difficulty extracting information from a geometric drawing.
Contenido relacionado
Mandelbrot set
Asymptote
Rational number