General relativity

The general theory of relativity or general relativity is a theory of the gravitational field and general reference systems, published by Albert Einstein in 1915 and 1916.
The name of the theory is due to the fact that it generalizes the so-called special theory of relativity and the principle of relativity for an arbitrary observer. The fundamental principles introduced in this generalization are the equivalence principle, which describes acceleration and gravity as different aspects of the same reality, the notion of the curvature of space-time, and the generalized covariance principle. The theory of general relativity proposes that the very geometry of space-time is affected by the presence of matter, resulting in a relativistic theory of the gravitational field. In fact, the theory of general relativity predicts that space-time will not be flat in the presence of matter and that the curvature of space-time will be perceived as a gravitational field.
Einstein's basic insight was to postulate that at a given point one cannot experimentally distinguish between a uniformly accelerated body and a uniform gravitational field. The general theory of relativity also made it possible to reformulate the field of cosmology.
Einstein expressed the purpose of the theory of general relativity to fully apply Ernst Mach's program of the relativization of all inertial effects, even adding the so-called cosmological constant to his field equations for this purpose. This real point of contact of Ernst Mach's influence was clearly identified in 1918, when Einstein distinguished what he baptized as Mach's principle (inertial effects derive from the interaction of bodies) from principle of general relativity, which is now interpreted as the principle of general covariance.
The German mathematician David Hilbert wrote and made public the covariance equations before Einstein, which resulted in not a few accusations of plagiarism against Einstein, but probably more so because it is a geometric theory (or perspective). It postulates that the presence of mass or energy "curves" space-time, and this curvature affects the trajectory of moving bodies and even the trajectory of light.
History
Shortly after formulating the theory of special relativity in 1905, Albert Einstein began to figure out how to describe gravitational phenomena with the help of new mechanics. In 1907 he embarked on an eight-year search for a new relativistic theory of gravity. After numerous detours and false starts, his work culminated on November 25, 1915 with the presentation to the Prussian Academy of Sciences of his article, which contained what are known today as "Field Equations". from Einstein & # 34;. These equations form the core of the theory and specify how the local density of matter and energy determines the geometry of space-time.
Einstein's field equations are nonlinear and very difficult to solve. Einstein used approximation methods in making the initial predictions of the theory. But already in 1916, the astrophysicist Karl Schwarzschild found the first non-trivial exact solution of Einstein's Field Equations, the so-called Schwarzschild Metric. This solution laid the foundation for the description of the final stages of a gravitational collapse, and the objects we know today as black holes. In the same year, the first steps towards the generalization of the Schwarzschild solution to electrically charged objects were taken, thus obtaining the Reissner-Nordström solution, now associated with the electrical charge of black holes.
In 1917, Einstein applied his theory to the universe as a whole, starting the field of relativistic cosmology. In line with contemporary thought, in which the universe was supposed to be static, he added a cosmological constant to his equations to reproduce that "observation". In 1929, however, the work of Hubble and others showed that our universe is expanding. This is easily described by the solutions found by Friedmann in 1922 for the cosmological expansion, which do not require a cosmological constant. Lemaître used these solutions to formulate the first version of the Big Bang models, in which our universe has evolved from a previous extremely hot and dense state. Einstein later declared that adding that cosmological constant to his equations was the biggest mistake of his life.
During that period, general relativity remained something of a curiosity among physical theories. It was clearly superior to Newtonian gravity, being consistent with special relativity and answering several effects not explained by Newtonian theory. Einstein himself had shown in 1915 how his theory was able to explain the advance of the anomalous perihelion of the planet Mercury without any arbitrary parameter. Similarly, in a 1919 expedition led by Eddington they confirmed the prediction of general relativity for the deflection of starlight by the Sun during the total solar eclipse of May 29, 1919, making Einstein instantly famous. However, this theory has entered the mainstream of theoretical physics and astrophysics developed roughly between 1960 and 1975, now known as the golden age of general relativity. Physicists began to understand the concept of a black hole, and to identify the manifestation of astrophysical objects such as quasars. Increasingly precise tests of the solar system confirmed the predictive power of the theory, and relativistic cosmology also became susceptible to direct observational tests.
Background
The explanatory successes of the theory of special relativity led to the acceptance of the theory by virtually all physicists. This led to the fact that before the formulation of general relativity there were two incompatible physical theories:
- The special theory of relativity, covariant in the sense of Lorentz, which adequately integrated electromagnetism, and which explicitly discards instant actions from a distance.
- The theory of Newton's gravitation, explicitly non-covariant, which adequately explained gravity through instant actions at a distance (concept of force at a distance).
The need to search for a theory that would integrate, as particular limit cases, the two previous ones required the search for a theory of gravity that would be compatible with the new relativistic principles introduced by Einstein. In addition to including gravitation in a theory of covariant formulation, there was another additional reason. Einstein had conceived the special theory of relativity as a theory applicable only to inertial reference frames, although it can really be generalized to accelerated systems without the need to introduce the whole apparatus of general relativity. Einstein's dissatisfaction with his belief that the theory was applicable only to inertial frames led him to search for a theory that would provide adequate physical descriptions for a fully general frame of reference.
This search was necessary, since according to spatial relativity no information can travel faster than light, and therefore there cannot be a causal relationship between two events linked by an interval of type space (space-like). However, one of the fundamental pillars of Newtonian gravity, the principle of action at a distance, supposes that the alterations produced in the gravitational field are transmitted instantly through space. The contradiction between both theories is evident, since assuming Newton's theses would imply the possibility that an observer would be affected by gravitational disturbances produced outside his light cone.
Einstein solved this problem by interpreting gravitational phenomena as simple alterations in the curvature of space-time produced by the presence of masses. From this it follows that the gravitational field, like the electromagnetic field, has an independent physical entity and its variations are transmitted at a finite speed in the form of gravitational waves. The presence of mass, energy or momentum in a certain region of the four-dimensional variety, causes the alteration of the metric coefficients, in a way whose detailed details will be analyzed in the following sections.
In this vision, gravitation would only be a pseudo-force (equivalent to the Coriolis force, or the centrifugal force) effect of having chosen a non-inertial reference system.
General principles
The essential characteristics of the theory of general relativity are the following:
- The general principle of covariance: the laws of physics must take the same mathematical form in all coordinate systems.
- The principle of equivalence or local invariance of Lorentz: the laws of special relativity (minikowski flat space) apply locally for all inertial observers.
- The curvature of space-time is what we observe as a gravitational field, in the presence of matter the geometry of space-time is not flat but curved, a particle in free movement inertial within a gravitational field follows a geodetic path.
Principle of Covariance
The principle of covariance is the generalization of the theory of special relativity, where it is sought that physical laws have the same form in all reference systems. The latter is equivalent to all reference systems being indistinguishable, and from the physical point of view equivalent. In other words, whatever the movement of the observers, the equations will have the same mathematical form and will contain the same terms. This was Einstein's main motivation to study and postulate general relativity.
The principle of covariance suggested that the laws should be written in terms of tensors, whose covariant and contravariant transformation laws could provide the "invariance" in a sought-after way, satisfying the physical principle of covariance.
The principle of equivalence
A fundamental milestone in the development of the theory of general relativity is the principle of equivalence, enunciated by Albert Einstein in 1912 and which its author described as "the happiest idea of my life». This principle assumes that a system that is in free fall and another that moves in a region of space-time without gravity are in a similar physical state: in both cases they are inertial systems.
Galileo distinguished between bodies with inertial motion (at rest or moving at constant speed) and bodies with non-inertial motion (subjected to accelerated motion). By virtue of Newton's second law (dating back to the work of the Spanish Dominican Domingo de Soto), all acceleration was caused by the application of an external force. The relationship between force and acceleration was expressed by this formula:
m=Fa{displaystyle m={frac {F}{a}}}}
where a is acceleration, F is force, and m is mass. The force could be of mechanical, electromagnetic or, of course, gravitational origin. According to Galileo's calculations, the gravitational acceleration of the bodies was constant and equaled 9.8 m/s2 on the Earth's surface. The force with which a body was attracted towards the center of the Earth was called weight. Obviously, according to the principles of classical mechanics, a body in free fall is not an inertial system, since it moves rapidly within the gravitational field in which it finds itself.
However, the theory of relativity considers that gravitational effects are not created by any force, but are caused by the curvature of space-time generated by the presence of matter. Therefore, a body in free fall is a (locally) inertial system, since it is not subjected to any force (because gravity has this character in general relativity). An observer located in an inertial system (such as a ship in orbit) does not experience any acceleration and is unable to discern whether or not he is going through a gravitational field. As a consequence, the laws of physics behave as if there were no gravitational curvature. Hence, the equivalence principle is also called Lorentz Local Invariance: In inertial systems, the principles and axioms of special relativity apply.
The equivalence principle also implies that observers located at rest on the Earth's surface are not inertial systems (they experience an acceleration of gravitational origin of about 9.8 meters per second squared, that is, " feel its weight").
| System | Is it inertial? (Principle of Equivalence) | Is it inertial? (Newtonian mechanics) |
|---|---|---|
| Body in free fall | Yes. | No. |
| Resting body on the ground surface | No. | Yes. |
| Planet orbiting around the sun | Yes. | No. |
| Ship rushing to the earth | Yes. | No. |
| Cohete taking off from a launch base | No. | No. |
Although classical mechanics takes into account the acceleration measured by an observer at rest with respect to the gravitational field (eg an astronomer); the Equivalence Principle, on the contrary, takes into account the acceleration experienced by an observer located in the system in question: any body that moves unrestricted by a gravitational field can be considered as an inertial system. This is the case of the planets that orbit around the Sun and the satellites that orbit around the former: the inhabitants of the Earth do not manage to perceive if we are getting closer to or further away from the Sun, nor if we are at aphelion or at perihelion, despite the enormous differences in solar gravity.
Gravity becomes, by virtue of the Principle of Equivalence, an apparent force, such as the centrifugal force and the Coriolis force: in these last two cases its appearance is due to the choice of an accelerating frame of reference (an observer located on the surface of a rotating sphere). In the case of gravity, we only perceive the apparent gravitational force when we choose a non-inertial reference system (at rest on the Earth's surface), but not when we place ourselves in another that is (a body in free fall).
Although the equivalence principle was historically important in the development of the theory, it is not a necessary ingredient of a theory of gravity, as evidenced by the fact that other metric theories of gravity, like the relativistic theory of gravitation dispense with the equivalence principle. It should also be noted that the equivalence principle is not fulfilled in the presence of electromagnetic fields, for example, a charged particle moving along a geodesic of any space-time will generally emit radiation, unlike a charged particle moving along of a geodesic of Minkowski space. That and other facts suggest that the equivalence principle, despite its historical equivalence, is not an essential part of a relativistic theory of gravitation.
The curvature of space-time
Albert Einstein's acceptance of the equivalence principle led him to a further discovery: the contraction or curvature of time as a consequence of the presence of a gravitational field, which was expressed in his 1911 article "On the influence of gravity on the propagation of light".
Suppose a photon emitted by a nearby star approaches Earth. By virtue of the law of conservation of the tetramomentum the conserved energy of the photon remains invariant. On the other hand, the equivalence principle implies that an observer located in the photon (which is an inertial system, that is, it is in free fall) does not experience any of the effects caused by the terrestrial gravitational field. From this it follows that the conserved energy of the photon does not change as a consequence of the action of gravity, nor does the frequency of light, since, according to the well-known formula of quantum physics, the energy of a photon is equal to its frequency v multiplied by Planck's constant h: E = hν.
Now, if the observations were made by an astronomer located on the surface of the Earth, that is, at rest with respect to its gravitational field, the results would be very different: the astronomer could verify how the photon, due to its fall towards the Earth, it progressively absorbs gravitational potential energy and, as a consequence of the latter, its frequency shifts towards the blue. The phenomena of energy absorption by photons in free fall and shift towards the blue are expressed mathematically by the following equations:
- Eorbs=Ecorne− − ≈ ≈ {displaystyle E_{obs}=E_{con}e^{-Phi }
- h.. rec=h.. eme− − ≈ ≈ {displaystyle hnu _{rec}=hnu _{em}e^{-Phi }}}
- .. rec=.. eme− − ≈ ≈ {displaystyle nu _{rec}=nu _{em}e^{-Phi },}
where Eorbs{displaystyle E_{obs},} is the energy measured by an observer at rest regarding the gravitational field (in this case an astronomer), ≈ ≈ {displaystyle Phi } the gravitational potential of the region where this is located, Ecorn{displaystyle E_{con}} the energy conserved from the photon, .. em{displaystyle nu _{em} emission frequency, .. rec{displaystyle nu _{rec}} is the frequency perceived by the observer (and run to blue) and h{displaystyle h} Planck's constant.
However, in the previous paragraph we have shown that the retained energy of the photon remains invariant. Therefore, how is it possible that this divergence exists between the results of the measurement of the energy obtained by astronomer (Eorbs{displaystyle E_{obs}}) and the preserved energy of the photon (Ecorn{displaystyle E_{con}}? The only way to resolve this contradiction is to consider that time slows as a result of the presence of a gravitational field. Thus, the aforementioned equation:
.. rec=.. eme− − ≈ ≈ {displaystyle nu _{rec}=nu _{em}e^{-Phi }
can be written like this:
cyclesΔ Δ torbs=cyclesΔ Δ teme− − ≈ ≈ {displaystyle {frac {mbox{ciclos}}{Delta t_{obs}}}}{frac {mbox{ciclos}{Delta t_{em}}}}}}}e^{-Phi }}
That is, the frequency is equal to the number of cycles taking place in a given period (usually a second). Where Δ Δ tem{displaystyle Delta t_{em} is the time measured by an observer located at an infinite distance from the mass body (and therefore does not experience the gravitational attraction of this), while Δ Δ torbs{displaystyle Delta t_{obs}} is the time measured by an observer under the influence of the gravitational field and resting on it (such as a person on the earth's surface). Hence it follows that near a massive body time slows, following these mathematical rules:
- Δ Δ tem=Δ Δ torbse− − ≈ ≈ {displaystyle Delta t_{em}=Delta t_{obs}e^{-Phi },}
- Δ Δ torbs=Δ Δ teme≈ ≈ {displaystyle Delta t_{obs}=Delta t_{em}e^{Phi },}
In a space-time singularity (such as those that exist inside black holes), the mass-matter density and the gravitational field tend to infinity, which causes the freezing of time and therefore the elimination of all kinds of dynamic processes:
limr→ → 0Δ Δ torbs=Δ Δ teme− − ∞ ∞ → → limr→ → 0Δ Δ torbs=0{displaystyle lim _{rto 0}Delta t_{obs}=Delta t_{em}e^{-infty }to lim _{rto 0}Delta t_{obs}=0}

The contraction of time due to the presence of a gravitational field was confirmed experimentally in 1959 by the Pound-Rebka-Snider experiment, carried out at Harvard University. Electromagnetic detectors were placed at a certain height and radiation was emitted from the ground. All the measurements that were made confirmed that the photons had undergone a redshift during their ascent through the Earth's gravitational field.
Today, the phenomenon of time contraction is of some importance within the framework of the GPS locator service, whose accuracy demands require extreme precision: A delay of 0.04 microseconds in the signal is enough for it to a positioning error of about 10 meters occurs. Hence, Einstein's equations have to be taken into account when calculating the exact location of a certain object on the earth's surface.
From a theoretical point of view, Einstein's 1911 paper was even more important. Well, the contraction of time also entailed, by virtue of the principles of special relativity, the contraction of space. Hence, it was inevitable from this moment on to rule out the existence of a flat space-time, and it was necessary to assume the curvature of the space-time variety as a consequence of the presence of masses.
In general relativity, phenomena that classical mechanics attributes to the action of the force of gravity, such as a free fall, the orbit of a planet or the trajectory of a spaceship, are interpreted as geometric effects of movement in a curved space-time. In fact a free particle in a gravitational field follows lines of minimum curvature through this time-curved space.
Finally, we can refer to the deflection of light rays as a consequence of the presence of a massive body, a phenomenon that gives rise to optical effects such as gravitational lenses or Einstein's rings.
Wavefront deviated. gravitational lens. Eddington's experiment.
Mathematical formulation and general considerations
Do not worry about your problems with mathematics; I assure you that mine are much greater.A. Einstein, in a letter to a nine-year-old girl.
Mathematically, Einstein conjectured that the geometry of the universe ceases to be Euclidean due to the presence of masses. Einstein modeled the universe as a type of curved spacetime by a pseudo-Riemannian manifold, and his field equations state that the sectional curvature of this manifold at a point is directly related to the energy-momentum tensor at that point.
This tensor is a measure of the density of matter and energy. Curvature "tells matter how to move", and reciprocally "matter tells space how to curve". In more precise terms, the trajectories of the particles are affected by the curvature, and the presence of many particles in a region noticeably alters the curvature. General relativity is distinguished from other alternative theories of gravity by the simplicity of coupling between matter and curvature.
Although there is still no quantum theory of gravity that incorporates both quantum mechanics and general relativity and proposes a gravitational field equation to replace Einstein's, few physicists doubt that a quantum theory of gravity will put general relativity in the proper limit, just as general relativity predicts the law of gravity in the non-relativistic limit.
The different tensors and scalars of general relativity
The Covariant Derivative


One of the essential concepts on which the whole theory of general relativity revolves is that of the covariant derivative (sometimes improperly called affine connection), which was defined for the first time by the Italian mathematician Tullio Levi-Civita and that can be considered both from a physical perspective and from another mathematical one.
From a physical point of view, the ordinary derivative of velocity is the acceleration of a body measured by an external observer at rest with respect to a gravitational field (for example, an astronomer located on the Earth's surface). In this case, the observer remains at a constant distance r from the center of mass, but not the observed object, which if we consider that it is in free fall, will progressively approach the origin of the gravitational field, and the external observer will detect that it has a constant acceleration g.
On the contrary, the covariant speed derivative (Du→ → dΔ Δ ){displaystyle left({frac {D{vec {u}}}{dtau }}{right)} or ► ► u→ → u→ → {displaystyle nabla _{vec {u}}{vec {u}}}}is the acceleration measured by a Automobile observer, that is, it is at rest regarding the body in free fall (e.g., the pilot of a plane in free fall or the crew members of a spacecraft with their engines off) and that unlike the ordinary drift, it will not detect any acceleration, unless the pilot lights the engines or some meteorite impacts it.
In short, the ordinary derivative is used to compute the ordinary acceleration of a body, while the covariant derivative is used to compute its inertial acceleration. According to Galilean and Newtonian mechanics, these two types of acceleration are identical, and on the basis of this axiom, new mechanical principles such as d'Alembert's Principle were developed. However, from Einstein's equivalence principle it follows that when a body is in free fall it has an ordinary acceleration that depends on the mass of the body on which it is falling, but its inertial acceleration is zero, unless some force is applied to it. another force. Hence, for Einstein it was absolutely necessary to introduce the concept of covariant derivative into his theory.
From a strictly mathematical point of view, the calculation of the covariant derivative takes place through a simple procedure. Firstly, the covariant partial derivative is computed and then it is generalized.
The ordinary derivative is applied exclusively on the components of a vector, while the covariant derivative is also applied on the bases of the vector space, since the perception of space-time will depend on the speed from the comobile observer:
- ► ► β β u→ → =▪ ▪ β β (uα α e→ → α α ){displaystyle nabla _{beta }{vec {u}}=partial _{beta }(u^{alpha }{vec {e}}_{alpha }}}}}
On this equation we proceed to apply the product (or Leibniz) rule,
- ► ► β β u→ → =(▪ ▪ β β uα α )e→ → α α +uα α (▪ ▪ β β e→ → α α ){displaystyle nabla _{beta }{vec {u}}=(partial _{beta} u^{alpha }){vec {e}}_{alpha }+u^{alpha }(partial _{beta }{vec {e}}_{alpha }}}}}}
At this point we introduced a new notation, the Christoffel symbolswhich can be defined as the component μ μ {displaystyle mu } partial derivative eα α {displaystyle e_{alpha }} concerning β β {displaystyle beta }: ▪ ▪ β β e→ → α α =Interpreter Interpreter α α β β μ μ e→ → μ μ {displaystyle partial _{beta }{vec {e}}_{alpha }Gamma _{alpha beta }^{mu }{vec {e}}{vec {e}}{mu }}. In this way:
- ► ► β β u→ → =(▪ ▪ β β uα α )e→ → α α +uα α Interpreter Interpreter α α β β μ μ e→ → μ μ {displaystyle nabla _{beta }{vec {u}}=(partial _{beta} }u^{alpha }){vec {e}}_{alpha }+u^{alpha }{Gamma _{alpha beta }{vec}{vec {e}}_{
We make an exchange of indices (μ μ {displaystyle mu } for α α {displaystyle alpha }) in the last term of the second member of the equation:
- ► ► β β u→ → =(▪ ▪ β β uα α )e→ → α α +Interpreter Interpreter μ μ β β α α uμ μ e→ → α α {displaystyle nabla _{beta }{vec {u}}=(partial _{beta} }u^{alpha }){vec {e}}_{alpha }{Gamma _{mu beta }{alpha }u^{alpha }{vec}{vec {e}}{alpha}}{alpha}}}
And with this we obtain the components of the covariant partial derivative of the velocity, which are equivalent to the expression in parentheses:
- ► ► β β u→ → =(▪ ▪ β β uα α +Interpreter Interpreter μ μ β β α α uμ μ )e→ → α α {displaystyle nabla _{beta }{vec {u}}=(partial _{beta} u^{alpha }+Gamma _{mu beta }^{alpha }u^{mu }{vec {e}}}{alpha }}}}
- ► ► β β uα α =▪ ▪ β β uα α +Interpreter Interpreter μ μ β β α α uμ μ {displaystyle nabla _{beta }u^{alpha }=partial _{beta }u^{alpha }+Gamma _{mu beta }{alpha }u^{mu }}
We generalize these components by multiplying them by the component β β {displaystyle beta } of tetravelocity (uβ β =dxβ β dΔ Δ {displaystyle u^{beta }={frac {dx^{beta }{dtau }}}}}) and obtain with it the covariant derivative of the speed:
- dxβ β dΔ Δ ► ► β β uα α =▪ ▪ β β uα α dxβ β dΔ Δ +Interpreter Interpreter μ μ β β α α uμ μ dxβ β dΔ Δ {displaystyle {frac {dx^{beta }{dtau}{nabla}{beta }u^{alpha }=partial _{beta }{alphad}{alphad}{dx{beta }{ }{dx }{beta ?
- ► ► u→ → uα α =duα α dΔ Δ +Interpreter Interpreter μ μ β β α α uμ μ uβ β {displaystyle nabla _{vec {u}}u^{alpha }={frac {du^{alpha }}{dtau }}}+Gamma _{mu beta }^{alpha }u^{mu }u^{beta }}}
Since for an inertial observer (e.g. a body in free fall) ► ► u→ → ua=0{displaystyle nabla _{vec {u}}u^{a}=0}This last equation takes the following form:
- 0=duα α dΔ Δ +Interpreter Interpreter μ μ β β α α uμ μ uβ β {displaystyle 0={frac {du^{alpha }}{dtau }{ gamma _{mu beta }^{alpha }u^{mu }u^{beta }}
- duα α dΔ Δ =− − Interpreter Interpreter μ μ β β α α uμ μ uβ β {displaystyle {frac {du^{alpha }}{dtau }}}{Gamma _{mu beta }^{alpha }u^{mu }u^{beta }}}
These formulas are called the equation of geodesic lines, and are used to calculate the gravitational acceleration of any body.

Beginner readers can collide with Christoffel's own definition of symbols. Finally, in the euclid space, the derivative of a base (e.g. ex{displaystyle e_{x}}) regarding another coordinate (Let's put and{displaystyle and}) is always zero, for the simple reason that the bases of both coordinates are orthogonal. However, this does not happen in the curved varieties, such as the surfaces of a cylinder or a sphere: In such cases, Christoffel symbols are not equal to zero, but are functions of those derived from the metric tensor. The mathematical relationship between these two mathematical magnitudes is expressed by the following equation:
- Interpreter Interpreter β β μ μ α α =12gα α σ σ (▪ ▪ μ μ gσ σ β β +▪ ▪ β β gσ σ μ μ − − ▪ ▪ σ σ gβ β μ μ ){displaystyle Gamma _{beta mu }^{alpha }={frac {1}{2}{2}g^{alpha sigma }(partial _{mu }{sigma beta }{beta }{beta }{beta
The Christoffel symbols are the main parameter that determines how big the existing degree of curvature is in a certain region and with their help we can know what the trajectory of a geodesic will be in a curved space. In the case of the space-time variety, the Theory of Relativity affirms that the curvature is caused by the presence of tetramomentum and therefore, the greater the density of matter existing in a certain region, the greater the values of the symbols. of Christoffel.
The principles of general covariance and least coupling
In a curved space-time, the laws of physics are modified by the Minimum Coupling Principle, which supposes that the mathematical equations by virtue of which they are expressed undergo the following modifications:
- The ordinary derivative is replaced by the covariant derivative.
- The Minkowski metric is replaced by a general formulation of the metric tensor.
- MIL MIL μ μ .. Δ Δ gμ μ .. (x){displaystyle eta _{mu nu }longrightarrow g_{mu nu }left(xright)}
- ▪ ▪ μ μ Δ Δ ► ► μ μ (x){displaystyle partial _{mu }longrightarrow nabla _{mu }left(xright)}
In this way, the Galilean equation of inertial systems is transformed by virtue of said principle into the relativistic equation of geodesic lines:
- ▪ ▪ β β uα α =0→ → ► ► β β uα α =0{displaystyle partial _{beta }u^{alpha }=0to nabla _{beta }u^{alpha }=0}
Law of conservation of energy:
- ▪ ▪ α α Tα α β β =0→ → ► ► α α Tα α β β =0{displaystyle partial _{alpha }T^{alpha beta }=0to nabla _{alpha }T^{alpha beta }=0}
However, by virtue of Christoffel's principle of symmetry of symbols, electromagnetic laws in general do not experience modifications due to gravitational curvature:
- Fα α β β =▪ ▪ α α Aβ β − − ▪ ▪ β β Aα α {displaystyle F_{alpha beta }=partial _{alpha }A_{beta}-partial _{beta }A_{alpha }}}}}}
- Fα α β β =► ► α α Aβ β − − ► ► β β Aα α {displaystyle F_{alpha beta }=nabla _{alpha }A_{beta}-nabla _{beta }A_{alpha }}}}}}
- Fα α β β =▪ ▪ α α Aβ β − − Interpreter Interpreter β β α α μ μ Aμ μ − − ▪ ▪ β β Aα α +Interpreter Interpreter α α β β μ μ Aμ μ {displaystyle F_{alpha beta }=partial _{alpha }A_{beta} }-Gamma _{beta alpha }{mu }A_{mu }-partial _{beta }A_{alpha } +Gamma _{alpha beta }{
- Interpreter Interpreter α α β β μ μ =Interpreter Interpreter β β α α μ μ {displaystyle Gamma _{alpha beta }^{mu }=Gamma _{beta alpha }^{mu }}
| Physical and Mathematical Law | Space-time plain | Space-time curved | Is there an alteration for the curvature? |
|---|---|---|---|
| Maintenance Act energy | ▪ ▪ α α Tα α β β =0{displaystyle partial _{alpha }T^{alpha beta }=0} | ► ► α α Tα α β β =0{displaystyle nabla _{alpha }T^{alpha beta }=0} | Yes. |
| Electromagnetic voltage | Fij=▪ ▪ iAj− − ▪ ▪ jAi{displaystyle F_{ij}=partial _{i}A_{j}-partial _{j}A_{i}}} | Fij=► ► iAj− − ► ► jAi=▪ ▪ iAj− − ▪ ▪ jAi{displaystyle F_{ij}=nabla _{i}A_{j}-nabla _{j}A_{i}A_{i}A_{j}-partial _{j}A_{i}}}} | No. |
| Maxwell equations | {displaystyle} | {displaystyle} | No. |
| Speed of light | c{displaystyle c} | c{displaystyle c} | No. |
| Equation of an inertial system | duα α dt=0{displaystyle {frac {du^{alpha}}{dt}}}=0} | ► ► u→ → u→ → =duα α dt+Interpreter Interpreter β β .. α α uβ β uμ μ =0{displaystyle nabla _{vec {u}}{vec {u}}}{frac {du^{alpha }}{dt}}}+Gamma _{beta nu }{^{alpha }u^{beta }u^{mu }=0} | Yes. |
| Acceleration | a=dx2dt2{displaystyle a={frac {dx^{2}{dt^{2}}}{dt^{2}} | aα α =d2xα α dΔ Δ 2{displaystyle a^{alpha }={frac {d^{2}x^{alpha }}{dtau ^{2}}}}} | Yes. |
| Volume | {displaystyle} | {displaystyle} | Yes. |
- Equation of geodetic lines
The Riemann tensor and the curvature of world lines
The measurement of the curvature of any manifold (be it space-time, a sphere or a saddle) is determined by the curvature tensor or Riemann tensor, which is a function of the Christoffel symbols and their first-order derivatives.
The Riemann tensor is of fundamental importance when calculating the deviation of two parallel lines in origin when they move through a curved surface. It is well known that in a flat variety parallel lines never intersect, however this rule does not apply in the case of curved surfaces of elliptical geometry. Suppose two travelers leave the equator heading north. In both cases, the angle that your ship's path makes with the Equator is initially 90°, so these are two parallel lines. However, as the travelers move north, their distance from each other becomes smaller and smaller until it becomes zero at the North Pole, which is where their travel paths intersect. To calculate the rate of approach between the two geodesics we use the following equation:
d2roga roga α α =Rβ β μ μ .. α α dxβ β roga roga μ μ dx.. {displaystyle d^{2}xi ^{alpha }=R_{beta mu nu }^{alpha }dx^{beta }xi ^{mu }dx^{nu }}
where dxβ β {displaystyle dx^{beta}} and dxμ μ {displaystyle dx^{mu}} represent the route from Ecuador of both geodetic lines and roga roga μ μ {displaystyle xi ^{mu }} the distance between them.
In space-time, which is also a curved manifold, things work in a similar way: the Riemann tensor determines the reciprocal acceleration between the world lines of two inertial systems (eg two asteroids colliding with each other). get progressively closer as a result of their mutual gravitational attraction). To calculate said acceleration, we apply the well-known formula again, modifying it slightly:
d2roga roga α α dΔ Δ 2=Rβ β μ μ .. α α uβ β roga roga μ μ u.. {displaystyle {frac {d^{2}xi ^{alpha }}{dtau ^{2}}}=R_{beta mu nu }^{alpha }u^{beta }{xi ^{mu }u^{nu }}}}
where dΔ Δ {displaystyle dtau } is an aphin parameter (local time) and uβ β {displaystyle u^{beta } and uμ μ {displaystyle u^{mu } are the quadrant cell vectors of both bodies that, according to the Minkowski scheme, geometrically equivalent to tangent vector fields to both universe lines.
All this connects us with what in Newtonian physics are called tidal forces, responsible for multiple astronomical phenomena and whose theoretical basis rests on the following approach: Suppose that a certain spaceship is falling at a black hole. It is evident that the bow of the ship experiences a stronger gravitational force than the stern, due to the simple fact that the former is closer than the latter to the event horizon. If the difference in accelerations between bow and stern is strong enough, the ship can become distorted and break forever.
The gravitational gradient is also responsible for the tidal cycle: The areas of the earth closest to the Moon experience a greater gravitational attraction than those furthest away from it, which causes seawater to accumulate in those areas of the Earth's surface that are aligned with the Moon.
In general relativity, the tidal acceleration is caused by the Riemann tensor. There is an almost natural correspondence between the Newtonian and relativistic equations. Indeed, the Newtonian equation used to compute the tidal forces is the following:
ai=≈ ≈ ,iiroga roga i{displaystyle a^{i}=Phi _{,ii}xi ^{i}}
where a It's tide acceleration, ≈ ≈ {displaystyle Phi } the gravitational potential roga roga {displaystyle xi } the distance between the two particles. The tide forces are determined by those derived from the second order of the gravitational potential.
From the relativistic point of view, the tidal forces are determined by the Riemann tensor, and if the region of space has a low quad-momentum density and a uniform curvature distribution, the components take approximately the following values:
- R0i0i≈ ≈ ≈ ≈ ,ii{displaystyle R_{0i0}^{i}approx Phi _{,ii}
- Rβ β μ μ .. α α ≈ ≈ 0{displaystyle R_{beta mu nu }^{alpha }approx 0} for the rest of the indexes
Rβ β μ μ .. α α =Interpreter Interpreter β β .. ,μ μ α α − − Interpreter Interpreter β β μ μ ,.. α α +Interpreter Interpreter β β .. σ σ Interpreter Interpreter σ σ μ μ α α − − Interpreter Interpreter β β μ μ ρ ρ Interpreter Interpreter ρ ρ .. α α ♪ Gamma ♪
In a frame of Lorentz, where the coefficients of Christoffel symbols are null and void but not their first derivatives, the formula for calculating the curvature tensor is simplified:
Rβ β μ μ .. α α =Interpreter Interpreter β β .. ,μ μ α α − − Interpreter Interpreter β β μ μ ,.. α α {displaystyle R_{beta mu nu }^{alpha }=Gamma _{beta numu }^{alpha }-Gamma _{beta munu }{alpha }}}}
If space-time is newtonian or quasi-newtonian (short density, non-relativist fluids) the only non-nul coefficients of Christoffel symbols are those corresponding to Interpreter Interpreter 00i{displaystyle Gamma _{00}^{i}}. We have therefore:
- Interpreter Interpreter 00i=≈ ≈ ,i{displaystyle Gamma _{00}^{i}=Phi _{,i}} otherwise Interpreter Interpreter β β μ μ α α =0{displaystyle Gamma _{beta mu }^{alpha }=0}
- R0i0i=Interpreter Interpreter 00,ii− − Interpreter Interpreter 0i,0i♪ Gamma ♪
- R0i0i=Interpreter Interpreter 00,ii♪ Gamma ♪
- R0i0i=≈ ≈ ,ii{displaystyle R_{0i0}^{i}=Phi _{,ii}}
Hence, it is very simple to deduce the classical equation from the relativistic one:
- d2roga roga idΔ Δ 2=R0i0iu0roga roga iu0→ → ai=≈ ≈ ,iiroga roga i{displaystyle {frac {d^{2}xi ^{i}}{dtau ^{2}}}}}=R_{0i0}{i}u^{0}xi ^{i}{o ^{i}to a^{i}=Phi _{,ii}xi ^{i}
As can be deduced from the previous paragraphs, in general relativity the tidal forces are determined by the Riemann tensor and the first derivatives of the Christoffel symbols. If these magnitudes have a non-zero value, the differential of the Christoffel symbols causes the dispersion of the geodesics corresponding to particles of a given fluid.
- ▪ ▪ Interpreter Interpreter β β μ μ α α I was.0{displaystyle partial Gamma _{beta mu }^{alpha }not =0}
- duα α dΔ Δ =− − Interpreter Interpreter μ μ .. α α uμ μ u.. {displaystyle {frac {du^{alpha }}{dtau }}{Gamma _{mu nu }^{alpha }u^{mu }u^{nu }}}}
Geodesics (inertial trajectories in space-time) are determined by the values of the Christoffel symbols. If these are constant, the particles of a fluid move uniformly, at the same speed and acceleration, and their distance from each other does not change. But if the components of the Christoffel symbols vary throughout a certain region, this entails the divergence of the world lines of the particles and the distortion of the fluid, to the extent that each of its constituent parts accelerates differently.
Tidal forces and the Riemann tensor are of fundamental importance in the formation and configuration of planetary systems, as well as in a multitude of astrophysical and cosmological processes. Take our own Solar System as an example: About 4.5 billion years ago, a molecular cloud reached sufficient density and compression to become a planetary system. Most of the material in the cloud precipitated out around the core, giving rise to the Sun. However, certain amounts of gas and dust continued to rotate in the form of an accretion disk, coalescing to form planetesimals. and later to planets.
However, in the area between Mars and Jupiter, the Riemann tensors corresponding to the masses of the Sun and Jupiter generated strong tidal forces that dispersed the world lines of the planetesimals located there, preventing them from aggregating together to give rise to a massive body. Planetesimals remained scattered in the form of an asteroid belt. This phenomenon that has just been described is not exclusive to our Solar System, but rather has been observed in a multitude of exoplanetary systems discovered from the early 1990s to the present, such as those shown in the illustrations in this section.
The tide forces also have some importance in the development of other astronomical phenomena such as type II supernovae, cosmic deflagrations that usually take place within the framework of double star systems. In fact, in binary systems it is common for a massive star to orbit around a white dwarf. If the size of the first one exceeds the Roche limit, the Riemann tensor component R0i0i{displaystyle R_{0i0}^{i}} generated by the mass of the white dwarf extracts material from the outer layers of his companion and precipitates it on the white dwarf, around which the material orbits forming an acreation disc. The plasma is subjected to enormous temperatures that cause the emission of X-rays and the emergence of periodic explosions known as type II supernovas.
The physical meaning of the Ricci tensor

According to the laplacian-newtonian theory of universal gravitation, a spherical mass of gas reduces its volume (as a result of the mutual attraction of its molecules) with an acceleration equivalent to 4Gπ π ρ ρ {displaystyle 4Gpi rho ;}:
Δ Δ V=4π π Gρ ρ {displaystyle Delta V=4pi Grho ;}
It is evident that said equation is not compatible with special relativity, for the reasons outlined above:
- The parameter ρ ρ {displaystyle rho ,}, which measures mass density, must be replaced by the power-tension tensor Tα α β β {displaystyle T^{alpha beta },}which remains invariable to the transformations of Lorentz and takes into account the gravitational effects of energy and pressure, and not only those of the mass.
- On the other hand, according to the theory of general relativity, gravitational effects are not caused by any kind of force at distance but by the curvature of space-time.
In this sense, it should be noted that in a time-space the acceleration of the volume is quantified by a specific geometric object, the Ricci tensor Rα α β β {displaystyle R^{alpha beta },}which can be defined as the coordinated acceleration of hypervolumen Русский Русский β β {displaystyle Pi _{beta }normal to the unit vector eβ β {displaystyle e_{beta },}. Thus, the component R00{displaystyle R^{00},} expresses the temporary acceleration of the three-dimensional volume:
R00=d2Русский Русский 0d(x0)2⇒ ⇒ R00=► ► 2V{displaystyle R^{00}={frac {d^{2}Pi _{0}{d(x^{0})^{2}}}{quad Rightarrow quad R^{00}=nabla ^{2}V}
The relationship between the metric tensor and the Ricci tensor is expressed through the so-called Ricci flow equation, which has the following form:
▪ ▪ tgα α β β =− − 2Rα α β β {displaystyle partial _{t}g_{alpha beta }=-2R_{alpha beta },}
According to this equation, the existence of positive values of the Ricci tensor implies the decrease over time of the coefficients of the metric tensor, and as a consequence of this, the decrease of the volumes in that region of the manifold. On the contrary, the presence of negative values in the Ricci tensor entails a progressive expansion of distances, surfaces and volumes.
For all that has been said, the energy-momentum and Ricci tensors made it possible to express the Poisson formula in a tensorial and covariant way, and that is why Einstein originally proposed the following equations of universe:
Rα α β β =4π π Gc2Tα α β β {displaystyle R^{alpha beta }={frac {4pi G}{c^{2}}}T^{alpha beta }}}
In general relativity, the Ricci tensor has the potential to represent those gravitational effects originated by the immediate and local presence of quadri-momentum, which are by far the most important on a small and large scale.
The Ricci tensor governs, then, most of the astrophysical processes that take place in the Cosmos: it constitutes a measure of the contraction of molecular clouds that give rise to the birth of stars and planets; quantifies the collapse of large stellar bodies and their conversion into white dwarfs, neutron stars, and black holes; and provides a measure of the expansion of the universe.
From the Ricci tensor, particularly from the form it takes in spherical gravitational fields (such as static stars), the so-called Hydrostatic equilibrium law is derived, which regulates the balance between pressure of the stellar fluid (which tends to expand the volume of the star) and the gravitational curvature (which contracts it). This balance is maintained practically throughout the life of the star and is only broken on two different occasions: 1) When the star becomes a red giant, in which case the effects of radiation pressure overwhelm those of the Ricci tensor, and as a result, the volume of the star expands until reaching a new equilibrium situation. 2) When the star runs out of fuel. There is then a drop in the pressure of the fluid, and the star either becomes a white dwarf, a neutron star, or collapses definitively becoming a black hole.
Einstein's Equations of the Universe
Einstein soon had to slightly modify his equations of the universe, as they were not compatible with the law of conservation of energy [Demonstration 1]. This forced Einstein to modify his equations of the Universe, which acquired his final form after the publication in 1915 of the article Application of the theory of general relativity to the gravitational field :
Rα α β β − − 12gα α β β R=8π π Gc4Tα α β β {displaystyle R^{alpha beta }-{frac {1}{2}{2}{alpha beta }R={frac {8pi G}{c^{4}}}}T^{alpha beta }}}
- ► ► β β Tα α β β =0{displaystyle nabla _{beta }T^{alpha beta }=0}
- Rβ β (μ μ .. σ σ )α α =0→ → Rβ β μ μ .. ,σ σ α α +Rβ β σ σ μ μ ,.. α α +Rβ β .. σ σ ,μ μ α α =0{displaystyle R_{beta (mu nu sigma)}^{alpha }=0to R_{beta musigma ^{alpha }+R_{beta sigma munu }{^{alpha }{alpha }{beta nu nu sigmamu ^}{
- ► ► β β (Rα α β β − − 12gα α β β R)=0→ → ► ► β β Rα α β β I was.0{displaystyle nabla _{beta }(R^{alpha beta }-{frac {1}{2}}}g^{alpha beta }R)=0to nabla _{beta }R^{alpha beta }{not =0}
- Rα α β β I was.kTα α β β {displaystyle R^{alpha beta }not =kT^{alpha beta }}
Where Rα α β β {displaystyle R^{alpha beta },} It's Ricci's tensor, gα α β β {displaystyle g^{alpha beta },} the metric tensor, R{displaystyle R,} Ricci's climbing, G{displaystyle G,} the constant universal gravitation and Tα α β β {displaystyle T^{alpha beta },} the energy-impulse tensor. The left member of the equation receives Einstein's generic tensor name, is represented with notation Gα α β β {displaystyle G^{alpha beta },} and meets the same conservation relationships as the tension-energy tensor:
► ► β β Gα α β β =► ► β β (Rα α β β − − 12gα α β β R)=0,Gα α β β =kTα α β β {displaystyle nabla _{beta }G^{alpha beta }=nabla _{beta left(R^{alpha beta }-{frac {1}{2}}}g^{alpha beta }Rright)=0,qquad G^{alpha beta α}{bbbbbbbk
Bearing in mind that the curvature scaler R{displaystyle R} is proportional to the trace of Einstein's tensor Gα α α α {displaystyle G_{alpha }^{alpha },}Einstein's universe equations can be reformulated as follows:
− − R=Gα α α α =8π π Gc4T{displaystyle -R=G_{alpha }^{alpha }={frac {8pi G}{c^{4}}T}
Rα α β β =8π π Gc4(Tα α β β − − 12gα α β β T){displaystyle R_{alpha beta }={frac {8pi G{c^{4}}}{left(T_{alpha beta }-{frac {1}{2}}}g_{alpha beta }Tright)}}
Application to perfect fluid
In a non-relativist fluid, like a nebula or a star of the main sequence, all the components of the energy-impulse tensor are null or of very little importance, except the element T00=ρ ρ c2{displaystyle T_{00}=rho c^{2},}which corresponds to mass density and which is the only one that contributes sensitively to gravitational attraction and the curvature of space-time. If we wish to measure the volume contraction produced by the mass-energy present in a given region, we must apply Einstein's universe equations:
Rα α β β =8π π Gc2(Tα α β β − − 12gα α β β T){displaystyle R_{alpha beta }={frac {8pi G{c^{2}}}}{left(T_{alpha beta }-{frac {1}{2}}}g_{alpha beta }Tright)}}
We now compute the values of R00{displaystyle R_{00}:
R00=8π π Gc2(T00− − 12g00T){displaystyle R_{00}={frac {8pi G}{c^{2}{2}}left(T_{00}-{frac {1}{2}}}{2}}}}}Tright}
After that we get:
T≈ ≈ c2T00→ → R00=4π π Gc2T00{displaystyle Tapprox c^{2}T_{00}to R_{00}={frac {4pi G}{c^{2}}}T_{00}}}}
Or:
- ► ► 2V=8π π G(ρ ρ − − ρ ρ c2− − 3P2c2)=4π π G(ρ ρ +3Pc2){displaystyle nabla ^{2}V={8pi G}left(rho -{frac {rho c^{2}-3P}{2c^{2}}}}}{2c^}}}}{2c^}{2cright)=4pi Gleft(rho +3{frac {P}{c}{c}{c^{c}{cright}}}}}}}}{cright
Where P{displaystyle P,} is the pressure of the fluid, which in general is very small compared to ρ ρ c2{displaystyle rho c^{2},}, so we have is a slight correction of the above-mentioned Newtonian formula. As we see, gravitational attraction is determined not only by mass-energy but also by pressure, although the contribution of this is c2{displaystyle c^{2}} lower than the first. Therefore, in the regions of space-time under low pressures and temperatures, such as nebulae or our Solar System, the mass is practically the only source of gravitational attraction and therefore the equations of Newtonian universal gravitation constitute a very good approximation of physical reality. On the other hand, in fluids subject to high pressures, such as the collapsing stars, the matter that rushes into the black holes or the jets that are expelled from the centers of the galaxies; in all of them the pressure can have some importance when computing the gravitational attraction and the curvature of space-time.
Application to electromagnetic fluid
In an electromagnetic fluid, the trace of the energy-momentum tensor is zero. As a consequence of this, Einstein's equations of the universe take the following form.
R00=8π π Gc2(T00− − 12g00T){displaystyle R_{00}={frac {8pi G}{c^{2}{2}}left(T_{00}-{frac {1}{2}}}{2}}}}}Tright}
T=0→ → R00=8π π Gc2(T00){displaystyle T=0to R_{00}={frac {8pi G}{c^{2}}}}{left(T_{00}right)}}
As we can see, the values of the Ricci tensor are just double those calculated for the powder solutions. This is what explains why the deflection of light rays is twice as great in the relativistic realm as in the Newtonian realm, and why the expansion of a Tolman cyclic universe (dominated by radiation) is slower than that of a Friedman's cyclic universe (dominated by matter).
The Weyl tensor
It is important to note that, placed in a four-dimensional space-time, the full curvature tensor contains more information than the Ricci curvature. This means that the equations of the previous field, with Λ = 0, do not completely specify the curvature tensor but a part of it, the Ricci tensor. The part of the curvature not specified by Einstein's equations coincides precisely with the Weyl tensor. That means that Einstein's equations do not fully specify the curvature tensor, nor the global shape of the universe.
The cosmological constant
Early on, Einstein appreciated that mathematically the right-hand side of his field equation could include a term proportional to the metric tensor without violating the principle of conservation of energy. Although he initially did not include the term, since it did not seem to have a reasonable physical interpretation, he later included it. This was because in his first attempts to find exact solutions to the field equations he considered what we now know as Einstein's stationary model. Einstein appreciated that this solution adequately explained the data available at his time, and corresponded to a static universe similar to the observed data. However, said solution was mathematically unstable, which did not seem to correspond to observable physical stability, and he realized that with the term proportional to the metric the solution could be similar but this time stable.
For this reason, Einstein introduced in his equations a term proportional to the metric tensor. Being the constant of proportionality precisely the cosmological constant. The work of several scientists (FLRW): Alexander Friedman, Georges Lemaître, Howard Percy Robertson and Arthur Geoffrey Walker, proved that non-stationary stable solutions exist without the term proportional to the cosmological constant. And although Einstein had initially rejected Friedman's work for describing an expanding universe that did not seem descriptively adequate to a universe he believed to be stationary, astronomer Edwin Hubble's redshift data seemed only explainable by an expanding universe model. This convinced Einstein that the FLRW solution was in fact correct and descriptively adequate and therefore the cosmological constant unnecessary.
Recently, the evidence of the acceleration of the expansion of the Universe has led to the reintroduction of the non-zero cosmological constant as one of the possible explanations of the phenomenon.
Summary
| Tenor | Notation | Physical significance |
|---|---|---|
| Regular derivative | duα α dt{displaystyle {frac {du^{alpha }}{dt}}}} | Acceleration measured by an external observer at rest |
| Covariant derivative | ► ► u→ → u→ → {displaystyle nabla _{vec {u}}{vec {u}}}} | Inertial acceleration measured by a common observer, located on the very line of universe of the observed body |
| Metric tension | gα α β β {displaystyle g_{alpha beta }} | Distance (or, in case, interval) between two points (events) of space(-time) |
| Power voltage tensor | Tμ μ .. {displaystyle T_{mu nu }} | Immediate presence of quadruple in a space-time region |
| Riemann Tensor | Rα α β β μ μ .. {displaystyle {R^{alpha }}_{beta mu nu }} | Mutual acceleration of two universe lines |
| Ricci Tensor | Rμ μ .. {displaystyle R_{mu nu }} | Acceleration of volume (3 dimensions) or hypervolumen (4 dimensions) |
| Climbing of Ricci | R{displaystyle R} | Acceleration of the surface that encloses the volume or hypervolumen |
| Weyl Tensor | Cβ β μ μ .. α α {displaystyle C_{beta mu nu }^{alpha }} | Tide forces generated by gravitational waves |
| Denomination | Development | Physical significance |
|---|---|---|
| Einstein universe equations | Recruitment of a fluid as a result of the immediate presence of a | |
| Equation of geodetic lines | Movement of an inertial system in space-time | |
| Geodetic deviation | Tide forces between two particles falling into the same gravitational field |
Solutions of Einstein's field equations
Mathematically Einstein's field equations are complicated because they constitute a system of 10 independent nonlinear differential equations. The complexity of such a system of equations and the associated difficulties in posing the problem as a well-defined initial value problem meant that for a long time there were only a handful of exact solutions characterized by a high degree of symmetry. Some hundreds of exact solutions of Einstein's equations are now known.
Historically, the first important solution was obtained by Karl Schwarzschild in 1915. This solution, later known as the Schwarzschild metric, represents the field created by a static and spherically symmetrical body. Said solution constitutes a very good approximation to the gravitational field within the solar system, which allowed subjecting the general theory of relativity to experimental confirmation, explaining previously unexplained facts, such as the advance of the perihelion of Mercury and predicting new facts later observed. as the deflection of light rays from a gravitational field. Furthermore, the peculiarities of this solution led to the theoretical discovery of the possibility of black holes, and a whole new area of cosmology related to them opened up. The study of gravitational collapse and black holes led to the prediction of spacetime singularities, a shortcoming that reveals that the theory of general relativity is incomplete.
Some other physically interesting solutions to Einstein's equations are:
- The Kerr metric that describes the gravitational field of a star in rotation. This solution under certain circumstances also contains a black Kerr hole.
- The metric of Friedman-Lemaître-Robertson-Walker is really a parametric set of solutions associated with the Big Bang theory that is able to explain the structure of the universe on a large scale and the expansion of it.
- The universe of Gödel, which in its original form does not seem to describe a realistic or similar universe to ours, but whose mathematically interesting properties constituted a stimulus to seek more general solutions of equations, to see if certain phenomena were peculiar to the simplest solutions.
On the other hand, the space-time used in the special theory of relativity, called Minkowski space, is itself a solution of Einstein's equations, which represents a space-time totally empty of matter.
Outside of the exact solutions and for comparative purposes with the gravitational field theory, the approximation for weak gravitational fields and the solutions in the form of gravitational waves are also interesting.
Nonlinearity
When Einstein formulated the equations of the universe of General Relativity in 1915, the German scientist initially thought that these equations were unsolvable due to their non-linear nature, which manifested itself both from a physical and from a physical point of view. another mathematician:
- In the strictly physical plane, the non-linearity of Einstein's field equations is derived from the mutual conditioning between the tetramomentum and the curvature of time space. Thus, mass density, contained in the coefficient T00{displaystyle T^{00}, causes a contraction (parameterized through R00{displaystyle R^{00}}) of the three-dimensional volume, which again alters the density of mass, and so on. This cyclic movement recalls the self-inductance of electromagnetism and does not usually have importance in low-intensity gravitational fields, but it must be taken into account in the calculation of the gravitational disturbances caused by a high local concentration of tetramomentum, as happens in the case of black holes or relativistic fluids. In a more intuitive way, the non-linearity of Einstein's equations can be thought from the physical point of view as follows: Given a distribution of matter, it will produce a curvature of space or "gravitational field", which contains energy. Given that E=mc2, such energy in turn will generate another curvature or "gravitational field", which in turn will contain some energy and so on. This is it. feedback between the source (matter) and the effect (curvature) is represented in the non-linear character of Einstein's equations.
- From a mathematical point of view, the left member of equality Rα α β β − − 12Rgα α β β =kTα α β β {displaystyle R_{alpha beta }-{frac {1}{2}Rg_{alpha beta }=kT_{alpha beta }}}} contains both linear and derivative functions of first and second order of the metric tensor gα α β β {displaystyle g_{alpha beta }}which makes it impossible to clear the coefficients of the latter from the values of the energy tensor momentum Tα α β β {displaystyle T_{alpha beta }}. It is not possible, therefore, to build a type function f:Tα α β β → → gα α β β {displaystyle f:T_{alpha beta }to g_{alpha beta }}.
Solutions for Spherical Coordinates: Exterior Field
To Albert Einstein's surprise, a few weeks after the publication of his field equations, an email arrived at his office from Karl Schwarzschild, a university professor who at the time was on the front lines of World War I, doing work ballistics for artillery units of the German army. This historic letter contained the first exact solutions of the equations of general relativity, which would be known to posterity under the generic name Schwarzschild Solution.
The principle on which the solution was pivoted was the following: Since the Principle of the General Covariance allowed to run the field equations of the general relativity in any coordinate system, Schwarzschild proceeded to calculate the values of the energy-moment tensors and Einstein in spherical space-temporal coordinates (θ θ ,φ φ ,r,t){displaystyle (thetaphir,t)}. The high degree of symmetry provided by the coordinate system, as well as the static character of the metric, allowed the direct integration of the set of differential equations. In the general case the metric tensor for a problem with spherical symmetry of the form:
(SEE)ds2=− − f(r)dt2+h(r)dr2+r2(dθ θ 2+without2 dφ φ 2){displaystyle ds^{2}=-f(r)dt^{2}+h(r)dr^{2}+r^{2}(dtheta ^{2}+sin ^{2}dphi ^{2}),}
For space, the outer part of a spherical body more specifically had:
f(r)=1h(r)=(1− − 2GMc2r){displaystyle f(r)={frac {1}{h(r)}}}}=left(1-{frac {2GM}{c^{2}r}}}}
Experimental verifications showed that the Schwarzschild metric describes with great precision what happens in static spherical systems, similar to the solar system.
Solutions for spherical coordinates: Stellar equilibrium
The equations of a field with spherical symmetry () also allow to study the curvature inside the massive stars. The result of this analysis is that for stars of the main sequence of the Hertzsprung-Russell diagram, the curvature originated by gravity is compensated by the pressure of the star matter. This compensation leads to a law of hydrostatic balance that makes the star, still subject to its own gravitational field, can hold its volume and density to constant levels for millions of years. Mathematically, the fact that the metric has a static character implies the values of the tensor Tα α β β {displaystyle T_{alpha beta }} stay stable in time. The law of hydrostatic balance that relates density and pressure in a spherical star is given by the equation of Tolman-Oppenheimer-Volkoff:
dPdr=− − G(P+ρ ρ c2r)(mc2+4π π r3Pc2r− − 2Gm){displaystyle {frac {dP}{dr}}}=-Gleft({frac {P+rho c^{2}}{r}}}{right)left({frac {mc^{2}+4pi r^{3}P}{c^{2}{2}r-2Gm}}}{right)}
Where:
- P(r),ρ ρ (r){displaystyle P(r),rho (r),} are pressure and density at a distance r the center of the star.
- m(r)=∫ ∫ 0rρ ρ (r! ! )4π π r! ! 2dr! ! {displaystyle m(r)=int _{0}^{r}rho ({bar {r}}}) 4pi {bar {r}}{2}d{bar {r}}}}}}}}} is the mass enclosed in a radio sphere r.
Solutions for Spherical Coordinates: Gravitational Collapse
Schwarzschild's solution made it possible to apply the postulates of general relativity to disciplines such as celestial mechanics and astrophysics, which meant a true revolution in the study of cosmology: barely six years after the publication of Einstein's work, the Russian physicist Aleksander Fridman introduced the concept of space-time singularity, defined as a point in space-time at which all the geodesics of the particles that had crossed the event horizon of a black hole converge. Under normal conditions, the curvature produced by the mass of bodies and particles is compensated by the temperature or pressure of the fluid and by electromagnetic forces, the study of which is the subject of fluid and solid state physics. However, when matter reaches a certain density, the pressure of the molecules is not capable of compensating for the intense gravitational attraction. The curvature of space-time and the contraction of the fluid increase with increasing speed: the logical end of this process is the emergence of a singularity, a point in space-time where the curvature and tetramomentum density are infinite.
Now, the physicist Subrahmanyan Chandrasekhar was the first to realize that gravity could be contained not only by mechanical forces, but also by a phenomenon of quantum origin which he called degeneracy pressure, derived from the Pauli exclusion principle and capable of supporting stars whose mass did not exceed the Chandrasekhar limit. These bold ideas cost its author dearly, who was publicly ridiculed by Sir Arthur Eddington during a congress of astronomers. However, Chandrasekhar's calculations turned out to be accurate, and served as the basis for the understanding of a stellar type whose physical nature was unknown until then: the white dwarf.
Harmonic Coordinate Approximations
Since it is not easy for many physical systems to obtain exact expressions for the solutions of Einstein's equations, theoretical physicists have developed fairly accurate approximations using power series. Among them, the most important work in harmonic coordinates and are called post-Newtonian approximation and approximation for weak gravitational fields.
By virtue of the principle of general covariance, already examined in previous sections, it is possible to make the universe equations of Einstein work in any type of coordinates, including harmonics, which are those in which the relationship is fulfilled. Interpreter Interpreter λ λ =gα α β β Interpreter Interpreter α α β β λ λ =0{displaystyle Gamma ^{lambda }=g_{alpha beta }Gamma _{alpha beta }^{lambda }=0} (such as in the case of Cartesian coordinates). It becomes necessary at this point to distinguish clearly between the concepts of plan space-time and harmony of a coordinate system: in a null curvature time-space, like Minkowski's time-space, it is possible to use non-armonic coordinates such as sphericals or cylindricals, without implying that the space is curved, since the curvature is an intrinsic quality of any variety and independent of our reference system.

For weak gravitational fields, such as those existing in interstellar space, it is advisable to use the so-called approximation for weak fields, which is, as we will see, very similar in structure to Poisson's formula Newtonian, although the differences with the latter are enormous.
Poisson's formula states that the laplatian of gravitational potential ≈ ≈ {displaystyle Phi } It's the same. 4Gπ π {displaystyle 4Gpi }:
► ► 2≈ ≈ =4π π Gρ ρ → → ≈ ≈ (x,t)=∫ ∫ VGρ ρ (x♫,t)rdV{displaystyle nabla ^{2}Phi =4pi Grho to Phi (x,t)=int _{V}{frac {Grho (x',t)}{r}}}{r
This formula has a serious drawback, and that is that it presupposes the principle of action at a distance: It does not take into account the delay in the measurement of the gravitational field carried out by a certain observer (let's say, an observer on earth) located at a certain distance. distance to the mass of the body that generates said gravitational field (for example, the Sun, located 8 light minutes from our planet).
Hence, one of the first attempts to reconcile the theory of Special Relativity and Universal Gravitation consisted of substituting the Laplacian of Poisson's formula for a d'Alembertian, one of whose solutions is, precisely, a retarded potential:
- deployment deployment 2≈ ≈ =4π π Gρ ρ → → ≈ ≈ (x,t)=∫ ∫ VGρ ρ (x♫,t− − rc)rdV{displaystyle Box ^{2}Phi =4pi Grho to Phi (x,t)=int _{V}{frac {Grho (x',t-{frac {r}{c}{r}}}}{r}}}
As we can see, the gravitational potential measured by the observer at time t, is proportional to the mass density of the stellar body observed at time t - r/c, where c is the speed of light, r is the distance between the observer and the object, and r/c is the delay, that is, the time it takes light to travel from the star in question to the observer.
However, general relativity is a metric theory of gravity, and explains gravitational phenomena in terms of metric disturbances. It is therefore convenient to introduce into our equation the pseudotensor hα α β β {displaystyle h_{alpha beta }}, which represents the deviation of the coefficients of the metric tensor from the Minkowski metric MIL MIL α α β β {displaystyle eta _{alpha beta }}. Applying the Newtonian boundary, by which virtue gα α β β {displaystyle g_{alpha beta }} equals 1+2≈ ≈ {displaystyle 1+2Phi }, we get the following result:
- gα α β β =MIL MIL α α β β +hα α β β {displaystyle g_{alpha beta }=eta _{alpha beta +}h_{alpha beta }}}
- hα α β β =2≈ ≈ → → deployment deployment 2hα α β β =8π π Gρ ρ {displaystyle h_{alpha beta }=2Phi to Box ^{2}h_{alpha beta }=8pi Grho }
- deployment deployment 2hα α β β =16π π G(Tα α β β − − 12gα α β β T){displaystyle Box ^{2}h_{alpha beta }=16pi G(T_{alpha beta }-{frac {1}{2}}}g_{alpha beta }T)}}
| Poisson Formula | ► ► 2≈ ≈ =4π π Gρ ρ {displaystyle nabla ^{2}Phi =4pi Grho } |
| Approximation for weak fields | deployment deployment 2hα α β β =16π π G(Tα α β β − − 12gα α β β T){displaystyle Box ^{2}h_{alpha beta }=16pi G(T_{alpha beta }-{frac {1}{2}}}g_{alpha beta }T)}} |
Big features, the replacement of the laplant ► ► 2{displaystyle nabla ^{2}} for the d'alembertian deployment deployment 2{displaystyle Box ^{2}} is required by the obligatory elimination of the principle of distance action; the use of the pseudotensor hα α β β {displaystyle h_{alpha beta }} instead of potential ≈ ≈ {displaystyle Phi } as a defining element of the gravitational field is a consequence of the metric character of the theory of general relativity; and finally, the elimination, on the right side of the equation, of the parameter ρ ρ {displaystyle rho } and its substitution by tensorial expression Tα α β β − − 12gα α β β T{displaystyle T_{alpha beta }-{frac {1}{2}{2}{alpha beta }T} is demanded by the principle of general covariance.
However, in the analysis of the evolution of astronomical systems such as the solar system or the one formed by double or tripole stars, the approximation for weak fields is not useful, since the use of the latter is restricted to areas of space- time with low tetramomentum density. In these cases, the post-Newtonian approximation is preferred, which, as its name indicates, dispenses with the use of the complex tensor calculus notation and describes the movement of celestial bodies using the mathematical concepts that Newton himself used in the time to describe the laws of mechanics and universal gravitation (vectors, gradients, etc.).
In the 18th and XIX, astronomers such as Laplace and Le Verrier had applied the postulates of Newtonian mechanics to the study of the evolution of the Solar System, obtaining very fruitful results: The precision of the astronomical calculations obtained had even allowed to foresee the existence of a planet never before observed by astronomers, Neptune. For this reason it is not surprising that when general relativity gained full recognition, astrophysicists developed an approximation that followed the Newtonian model in its structure and that was easily applicable both by astronomers and by computers.
According to the classical theory of gravitation, the acceleration of a freely falling body is the negative gradient of the gravitational potential:
- a=− − ► ► φ φ {displaystyle a=-nabla phi }
As has already been advanced in previous sections, this formula presupposes the assumption of the Newtonian principle of distance action, contrary to the postulates of Special Relativity, and also does not take into account the gravitational effects generated by energy and momentum. La Post-Newtonian approach soslaya these drawbacks by introducing two new potentials: the potential END END {displaystyle psi }which constitutes a second degree approach to the potential φ φ {displaystyle phi } and potential γ γ {displaystyle zeta }, derived from the presence of momentum in the fluid.
| Notation | Algebraic expression | Physical significance |
|---|---|---|
| φ φ {displaystyle phi } | φ φ =− − ∫ ∫ Gρ ρ rdV{displaystyle phi =-int {frac {Grho }{r}{r}}dV} | Newtonian potential (mass density) |
| END END {displaystyle psi } | END END =[chuckles]14π π ▪ ▪ 2φ φ ▪ ▪ t2+G(Ek+Ep)+G(Ek)]{displaystyle psi =[{frac {1}{4pi }}{frac {partial ^{2phi }{partial t^{2}}}}}}+G(E_{k}+E_{p})+G(E_{k})}}}} | Retardo of Newtonian potential, energy density |
| γ γ {displaystyle zeta } | γ γ =− − 4G∫ ∫ PrdV{displaystyle zeta =-4Gint {frac {P}{r}}dV} | Potential derived from momentum |
The equations of motion would be reformulated as follows:
- a=− − ► ► (φ φ +2φ φ 2c2+END END )− − 1c▪ ▪ γ γ ▪ ▪ t+vc× × (► ► × × γ γ )+3c2v▪ ▪ φ φ ▪ ▪ t+4c2v(v⋅ ⋅ ► ► )φ φ − − v2c2► ► φ φ {cHFFFFFF}{cHFFFFFF}{cHFFFFFF}{cHFFFFFF}{cHFFFFFF}{cHFFFFFFFF}{cHFFFFFFFFFF}{cHFFFFFFFF}{cHFFFF}{cHFFFFFF}{cHFFFFFFFF}{c}{cHFFFFFFFFFFFFFFFFFFFFFFFFFF}{c}{cH00}{c}{cH00}{cHFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFF00}{cHFFFFFFFFFFFFFFFFFFFF00}{c}{cHFFFFFFFFFFFF00}{cH00}{cH00}{c}{cHFFFFFFFFFFFFFFFF00}{cHFFFFFFFFFFFF00}{c}{cH00}{cH00}{c}{cH
- a=− − ► ► φ φ +MIL MIL {displaystyle a=-nabla phi +eta }
- MIL MIL =− − ► ► (2φ φ 2c2+END END )− − 1c▪ ▪ γ γ ▪ ▪ t+vc× × (► ► × × γ γ )+3c2v▪ ▪ φ φ ▪ ▪ t+4c2v(v⋅ ⋅ ► ► )φ φ − − v2c2► ► φ φ {cHFFFFFF}{cHFFFFFF}{cHFFFFFF}{cHFFFFFF00}{cHFFFFFF00}{cHFFFFFF00}{cHFFFFFF00}{cHFFFFFF00}{cHFFFF00}{cHFFFFFFFFFF00}{cHFFFFFFFFFF00}{cHFFFFFFFFFFFFFFFFFFFF00}{cH00}{cH00}{cH00}{cHFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFF00}{cH00}{cHFFFFFF00}{c}{cH00}{cHFFFFFFFFFFFFFFFFFFFFFFFF00}{c}{cH00}{c}{cHFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFFF
Solution of the relativistic total force
In general relativity, the effective gravitational potential energy of an object of mass m moving around a central massive body M is given by
Uf(r)=− − GMmr+L22mr2− − GML2mc2r3{displaystyle U_{f}(r)=-{frac {GMm}{r}}}}{L^{2mr^{2}}}{2mr^{2}}}}{frac {GML^{2}}{mc^{2}r^{3}}}}}}}
Then a conservative total force can be obtained as
Ff(r)=− − GMmr2+L2mr3− − 3GML2mc2r4{displaystyle F_{f}(r)=-{frac {GMm}{r^{2}}}}{frac {L^{2}}{mr^{3}}}}}}}-{frac {3GML^{2}}{mc^{2}r^{4}}}}}}}}
where L is angular momentum. The first term represents the Newtonian gravitational force, which is described by the inverse square law. The second term represents the centrifugal force in circular motion. The third term is related to the Coriolis force in the rotating frame of reference, which includes the inverse of the distance to the fourth power.
Solutions related to Universe models
There are a certain number of exact solutions of the equations that describe a complete universe and therefore can be considered cosmological models, among them are:
- Metric of Friedman-Lemaître-Robertson-Walker, which describes a type of homogeneous, isotropic and expanding universe and can be considered a first approximation of the form of our large-scale universe.
- Gödel Universe, obtained by the mathematician Kurt Gödel represents a homogeneous and isotropical universe with matter in rotation. Although it is not considered to describe a universe similar to ours it has the important property to contain closed temporary curves that represent a counterintuitive example where an observer can travel to his own past without violating any known physical law.
Predictions of general relativity
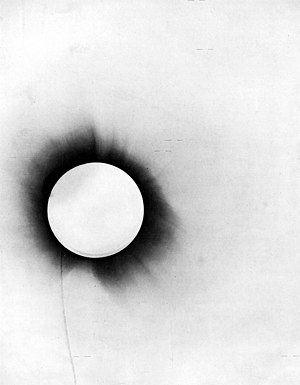
It is considered that the theory of general relativity was verified for the first time in the observation of a total eclipse of the Sun in 1919, carried out by Sir Arthur Eddington, in which it was revealed that the light coming from distant stars it curved as it passed close to the Sun's gravitational field, altering the apparent position of stars near the Sun's disk. Since then many other experiments and applications have proven the predictions of general relativity. Some of the predictions include:
Gravitational Effects
- Gravitational deviation of light to red in the presence of fields with intense gravity: The frequency of light decreases by passing through a region of high gravity. Confirmed by the experiment of Pound and Rebka (1959).
- Gravitational dilation of time: The clocks placed in conditions of high gravity mark the time more slowly than clocks placed in an environment without gravity. Experimentally demonstrated with atomic clocks located on the Earth's surface and the clocks in orbit of the Global Positioning System (GPS). Also, although it is very small time intervals, the different tests performed with planetary probes have given values very close to those predicted by general relativity.
- Shapiro Effect (gravitational expansion of temporary defases): Different signs crossing an intense gravitational field need more time to go through the field.
- Orbital decay due to the emission of gravitational radiation. Observed in binary pulpits.
- Geodetic Precession: Due to the curvature of space-time, the orientation of a rotational gyroscope will change over time. This was successfully verified in May 2011 by the Gravity Probe B satellite.
Rotary Effects
This implies the behavior of space-time around a rotating massive object.
- Frame friction. An object in full rotation will drag with it into space-time, causing the orientation of a gyroscope to change over time. For a spacecraft in polar orbit, the direction of this effect is perpendicular to geodetic precision.
- The principle of strong equivalence: even objects that gravitate around themselves will respond to an external gravitational field in the same way that a test particle would.
Solution to galactic rotation curves
An alternative solution based on general relativity proposed by Adrián Cornejo, which considers the gas velocity patterns in simulated galaxies, according to the quasi-stationary density wave theory that characterizes spirals as patterns of rigid rotation and of long-lived (i.e., stable spirals), and the hypothesis that spiral galaxies would rotate stably mostly as a rigid solid body, consistent with rigid-solid kinematics, calculated the velocity of some Sa-type spiral galaxies (according to the morphological classification of galaxies), plotting the rotation curves and obtaining (without considering dark matter) curves very close to the rotation curves known from observations. The equation results from equating the centrifugal force with the force that is added to Newtonian mechanics in the relativistic solution (which is the force inversely proportional to the fourth power of the distance r, related to the Coriolis force in the non-inertial reference frame), analogous to how the orbital velocity of planets is derived by Newtonian mechanics. In this way, we have the Coriolis force in the rotating system, which is a force that in Newtonian mechanics is not considered in a rotating reference system. With what we have in the rotating system the force that, in another way, seeks to add through dark matter to adjust to the observed rotation speeds. The equation as a function of angular momentum is given as
v=(3GM∙ ∙ J2c2r3Mg2)1/2=(3GM∙ ∙ j2c2r3)1/2{displaystyle v=left({frac {3GM_{bullet }J^{2}}{c^{2}r^{3}M_{g}{2}}}}{2}}}}{1/2}=left({3GM{bullet }{2}{c^{2}{1⁄2}{1⁄2}r{3⁄2}}}}{3⁄2}}}}}}}{
where c is the speed of light, J is the angular momentum of the system, j is the specific relative angular momentum, M● is the mass of the galactic nucleus and Mg is the mass of the galaxy. This formula only applies to Sa-type spiral galaxies due to their circular symmetry, since for other types of spiral galaxies with different morphology their different geometry and dynamics would have to be considered. Although to date there are not many documented observations of the angular momentum of spiral galaxies, and the estimates that do have are mainly derived from models using the concept of dark matter, the angular momentum of each galaxy can be estimated from the moment specific relative angular, given as h = L/m, or in this case as j = J/Mg. The proper angular momentum for each case can be verified with more detailed observations of spiral galaxies.
Other effects
- Gravitons: According to the quantum theory of fields, gravitational radiation must be composed of so-called gravitons. General relativity predicts that these will be particles of sphin 2. They haven't been observed yet.
Checks
The theory of general relativity has been confirmed in numerous ways since its inception. For example, the theory predicts that the world line of a ray of light bends in the vicinity of a massive object like the Sun. The first empirical test of the theory of relativity was in this regard. During the eclipses of 1919 and 1922, scientific expeditions were organized to make these observations, including the Arthur Eddington expedition. The apparent positions of the stars were then compared with their apparent positions some months later, when they appeared at night, far from the Sun. Einstein predicted an apparent position shift of 1.745 arcseconds for a star located just at the edge of the Sun., and smaller and smaller displacements of the more distant stars. His calculations of the bending of light in the presence of a gravitational field were shown to be accurate. Similar measurements of the deflection of radio waves from distant quasars have been made in recent years using radio interferometers. The measurements yielded results that agreed to within 1% of the values predicted by general relativity.
Another confirmation of general relativity is related to the perihelion of the planet Mercury. It had been known for years that perihelion (the point at which Mercury is closest to the Sun) revolves around the Sun once every three million years, and that movement could not be fully explained by classical theories. In contrast, the theory of relativity does predict all aspects of motion, and recent radar measurements have confirmed that the actual data match the theory to within 0.5%.
There have been many other tests of the theory, and so far they all seem to confirm it. Practically with the most recent test of the Gravity Probe B satellite, the theory could be considered as a law.
Practical applications
The clocks in GPS satellites require synchronization with those located on the ground, for which the general theory of relativity and the special theory of relativity must be taken into account. If the effect of the speed of the satellite and its gravity relative to an observer on the ground on time were not taken into account, the satellite clock would gain 38 microseconds per day (without correction, your clock would lose one day). 7 microseconds as a consequence of the speed and would advance 45 microseconds due to the effect of gravity), which in turn would cause errors of several kilometers in the determination of the position. It can be considered another verification of both theories.
Relation to other physical theories
In this part, classical mechanics and special relativity are intertwined because general relativity in many ways is intermediate between special relativity and quantum mechanics.
Subject to the principle of least coupling, the physical equations of special relativity can be converted to their general relativity equivalent by replacing the Minkowski metric (ηab) with the relevant spacetime metric (gab) and replacing any normal derivatives with covariant derivatives.
Inertia
In both quantum mechanics and relativity, space, and later space-time, was assumed to be flat. In the language of tensor calculus, this meant that Rabcd = 0, where Ra bcd is the Riemann tensor of curvature. Additionally, the coordinate system was assumed to be a Cartesian coordinate system. These restrictions allowed the inertial motion to be described mathematically as:
x! ! a=0,{displaystyle {ddot {x}}^{a}=0,} where
- xa It's a position vector,
- ! ! =▪ ▪ /▪ ▪ Δ Δ {displaystyle {dot {}}=partial /partial tau }and
- Δ It's own time.
Note that in classical mechanics, xa is three-dimensional and τ ≡ t, where t is a time coordinate.
In general relativity, if these constraints are used in the form of space-time and the coordinate system, they will be lost. This was the main reason why a different definition of inertial motion was needed. In special relativity, inertial motion occurs in Minkowski space as parameterized by proper time. This is generalized to curved spaces mathematically by the equation of geodesics:
x! ! a+Interpreter Interpreter abcx! ! bx! ! c=0,{displaystyle {ddot {x}}^{a}+{Gamma ^{a}_{bc},{dot {x}{x}{b}{dot {x}}}}}{,{dot {x}}{x}}}}{,{cddot {x}}{x}}{x}}{x}}}}{, {c}}{ where
- Interpreter Interpreter abc{displaystyle {Gamma ^{a}_{bc}} is a symbol of Christoffel (otherwise known as Levi-Civita connection).
Like x It is a rank one tensor, these equations are four and each one is describing the second derivative of a coordinate with respect to time itself. (In the Minkowski metric of special relativity, the connection values are all zero. This is what makes geodetic equations of general relativity x! ! a=0{displaystyle {ddot {x}}^{a}=0} for the flat space of special relativity).
Gravitation
In gravitation, the relationship between Newton's theory of gravity and general relativity is governed by the correspondence principle: general relativity has to produce the same results, just as gravity does in cases where physics Newtonian has been shown to be accurate.
Around symmetrically spherical objects, Newton's theory of gravity predicts that the other objects will be accelerated towards the center by the law:
F=− − GM.mr2r^ ^ {displaystyle mathbf {F} =-{frac {GM.m}{r^{2}}}}{mathbf {hat {r}}} }
Where: M: mass generated by the gravitational field, and m is the mass of the body that is attracted.
- r{displaystyle r,}is the distance to the object attracted, and
- r^ ^ {displaystyle mathbf {hat {r}} } is a unit vector identifying the direction to the mass object.
In the weak field approximation of general relativity there must be an acceleration at identical coordinates. In the Schwarzschild solution, the same acceleration of the force of gravity is obtained when the constant of integration is equal to 2m (where m = GM/c2).
Electromagnetism
Electromagnetism posed a fundamental obstacle to classical mechanics, because Maxwell's equations are not invariant under Galilean relativity. This created a dilemma that was resolved by the advent of special relativity. In tensor form, Maxwell's equations are:
▪ ▪ aFab=(4π π /c)Jb{displaystyle partial _{a},F^{,ab}=(4pi /c),J^{,b}}}and
▪ ▪ aFbc+▪ ▪ bFca+▪ ▪ cFab=0{displaystyle partial ^{a},F^{,bc}+partial ^{b},F^{,ca}+partial ^{c},F^{,ab}=0}
Where:
- Fab{displaystyle F_{ab},}It's the electromagnetic field tensor, and
- Ja{displaystyle J_{a},}It's a quadrilateral.
The effect of an electromagnetic field on a charged object of mass m is then:
dPadΔ Δ =qmPbFab{displaystyle {frac {dP^{a}{dtau }}}{{{frac {q}{m}{b}F^{ab}}}}
Where
- Pa{displaystyle P^{a},} is the quadruple of the loaded object.
In general relativity, Maxwell's equations become
► ► aFab=(4π π /c)Jb{displaystyle nabla _{a},F^{,ab}=(4pi /c),J^{,b}}} and
► ► aFbc+► ► bFca+► ► cFab=0{displaystyle nabla ^{a},F^{,bc}+nabla ^{b},F^{,ca}+nabla ^{c},F^{,ab}=0}.
The equation for the effect of the electromagnetic field remains the same, although changing the metric will modify your results. Note that when integrating this equation for accelerated charges the usual assumptions do not hold (as they imply that a charge held in a gravitational field must behave as if it were uniformly accelerated, which shows that a uniformly accelerated charge cannot radiate).
Conservation of energy-momentum
In classic mechanics, energy conservation and momentum are handled separately. In the special relativity, energy and momentum are united in the quadruple and the power tensors. For any physical interaction, the energy-impulse tensor Tab{displaystyle {T_{a}}{b}}} meets the following local conservation law:
▪ ▪ bTab=0{displaystyle partial _{b},{T_{a}}}{b}=0}
In general relativity, this relationship is modified to account for curvature, becoming:
► ► bTab=▪ ▪ bTab+Interpreter Interpreter bcbTac+Interpreter Interpreter cabTcb=0{displaystyle nabla _{b},{T_{a}}}{b}=partial _{b},{T_{a}}{b} +{Gamma ^{b}}}{b}{cb}{,{t_{a}}}}{,{cb_{a}{c}}{,{cb_{cb}}}}{,{,{
where ∇ here represents the covariant derivative.
Unlike classical mechanics and special relativity, in general relativity it is not always possible to clearly define total energy and momentum. This often causes confusion in time-dependent space-times, where there are no temporal killing vectors, which do not appear to conserve energy, even though the local law is always satisfied (See Arnowitt, Deser and Misner energy).
Transition from special relativity to general relativity
The theory of special relativity presents Lorentz covariance; this means that, as formulated, the laws of physics are written in the same way for two observers who are inertial. Einstein estimated, inspired by the equivalence principle, that a theory was necessary that presented one for which a generalized covariance principle would hold, that is, in which the laws of physics were written in the same way for all possible observers, were these inertial or not; that led him to look for a general theory of relativity. In addition, the fact that the theory of relativity itself was incompatible with the principle of action at a distance made him understand that he also needed this general theory to incorporate an adequate description of the gravitational field.
Today we know that Einstein considered that the theory of relativity was only applicable to strictly inertial reference frames, although Logunov has proven within the framework of the relativistic theory of gravitation that in fact fixed an inertial observer or not, any other that moves with uniform velocity with respect to the first will write the physical laws in the same way. Thus proving that special relativity is in fact more general than Einstein believed at the time. In addition, Logunov's work proves that whenever space-time is flat it can be established for each observer there is a decaparametric group of coordinate transformations that generalizes the properties of the Lorentz group for non-inertial observers.
The principle of geometrization and the principle of equivalence were the cornerstones on which Einstein based his search for a new theory, after having failed in the attempt to formulate a relativistic theory of gravitation from a gravitational potential. Nordström's scalar theory of gravitation and the geometric interpretation of it derived by Adriaan Fokker (1914), Hendrik Lorentz's doctoral student, led Einstein to be able to relate the energy-momentum tensor to the Ricci scalar curvature of a space-time with metric:
gα α β β =φ φ MIL MIL α α β β {displaystyle g_{alpha beta }=phi eta _{alpha beta },}
which involved the metric of flat space-time and a scalar field related to the gravitational field. Overcoming the shortcomings of Nordström's theory of scalar gravitation led Einstein to formulate the correct field equations.
Contenido relacionado
Polyoxometalate
Leon Foucault
Cyclic Redundancy Check

















































































































































































![psi =[{frac {1}{4pi }}{frac {partial ^{2}phi }{partial t^{2}}}+G(E_{k}+E_{p})+G(E_{k})]](https://wikimedia.org/api/rest_v1/media/math/render/svg/fc2a0b8ed0d0570ba09f8eba324affe489e9df00)


























