Gamma function

AjustarCompartirImprimirCitar

Gamma function in the real axis

Gaumma function module in complex plane

In mathematics, the gamma function (denoted as ${displaystyle Gamma (z)}$ Where ${displaystyle Gamma }$ It's the Greek letter. gamma in capital), it is an application that extends the concept of factorial to real and complex numbers. The notation was proposed by Adrien-Marie Legendre. If the real part of the complex number $z$ is positive, then the integral

{displaystyle Gamma (z)=int _{0}^{infty }t^{z-1}e^{-t};dt}

converges absolutely; this integral can be extended to the entire complex plane, except to negative integers and to zero. Yeah. ${displaystyle nin mathbb {Z} ^{+}}$ then.

{displaystyle Gamma (n)=(n-1)!}

which shows us the relation of this function to the factorial. In fact, the gamma function extends the concept of factorial to any complex value of $z$ . The gamma function appears in several probability distribution functions, so it is quite used both in probability and in statistics and in combination.

Definition

The gamma function in the complex plane

Notation ${displaystyle Gamma (z)}$ is due to Legendre. If the real part of the complex number $z$ is strictly positive $0right)}" xmlns="http://www.w3.org/1998/Math/MathML">(Re(z)▪0){displaystyle left({text{Re}(z)/20050right)}0right)}" aria-hidden="true" class="mwe-math-fallback-image-inline" src="https://wikimedia.org/api/rest_v1/media/math/render/svg/d9c2b4ef61a93b0e68922fd7d29e027490241b4f" style="vertical-align: -0.838ex; width:11.711ex; height:2.843ex;"/>$ , then the whole

{displaystyle Gamma (z)=int _{0}^{infty }t^{z-1}e^{-t},dt}

converges absolutely and is known as a Euler integral of the second order. Using integration by parts, we obtain the following property:

{displaystyle {begin{aligned}Gamma (z+1)&=int _{0}^{infty }t^{z}e^{-t}dt\&=-t^{z}e^{-t}{bigg |}_{0}^{infty }+int _{0}^{infty }zt^{z-1}e^{-t}dt\&=zint _{0}^{infty }t^{z-1}e^{-t}dt\&=z;Gamma (z)end{aligned}}}

We can get ${displaystyle Gamma (1)}$ :

{displaystyle {begin{aligned}Gamma (1)&=int _{0}^{infty }t^{1-1}e^{-t}dt\&=int _{0}^{infty }e^{-t}dt\&=lim _{bto infty }-e^{-t}{bigg |}_{0}^{b}\&=0-(-1)\&=1end{aligned}}}

Having to ${displaystyle Gamma (1)=1=0!}$ and ${displaystyle Gamma (n+1)=nGamma (n)}$ then.

{displaystyle Gamma (n+1)=Gamma (1)left(prod _{k=1}^{n}kright)=left{{begin{array}{ll}Gamma (1)=1=0!,&n=0\1cdot 2cdot 3dotsb n=n!,&nin mathbb {N} ^{*}end{array}}right.}

for all natural $n$ .

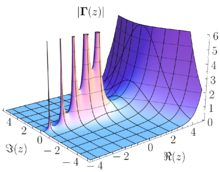
The gamma function is a meromorphic function ${displaystyle zin mathbb {C} }$ with simple poles in ${displaystyle z=-n,,(n=0,,1,,2,,3,,dots)}$ and waste ${displaystyle operatorname {Res} (Gamma (z),-n)={frac {(-1)^{n}}{n!}}}$ . These properties can be used to extend ${displaystyle Gamma (z)}$ from its initial definition to the entire complex plane (except the points in which it is singular) by analytical continuation.

Alternate definitions

Definition of Euler as an infinite product

For everything $m$ verified

{displaystyle lim _{nto infty }{frac {n!(n+1)^{m}}{(n+m)!}}=lim _{nto infty }{(n+1)^{m} over (n+1)cdots (n+m)}=1}

Yeah. $m$ is not an integer then it is not possible to say if the previous equation is valid because in this section the factorial function has not yet been defined for integers. However, we can obtain an extension of the factorial function for not integers demanding that this relationship remain valid for an arbitrary complex number $z$ :

{displaystyle lim _{nto infty }{frac {n!(n+1)^{z}}{(n+z)!}}=1}

By multiplying both sides by ${displaystyle z!}$ obtained

{displaystyle {begin{aligned}z!&=lim _{nto infty }n!;{frac {z!}{(n+z)!}}(n+1)^{z}\&=lim _{nto infty }(1cdots n){frac {1}{(z+1)cdots (z+n)}}left({frac {2}{1}}cdot {frac {3}{2}}cdots {frac {n}{n-1}}{frac {n+1}{n}}right)^{z}\&=prod _{n=1}^{infty }left[{frac {1}{1+{frac {z}{n}}}}left(1+{frac {1}{n}}right)^{z}right]end{aligned}}}

This infinite product converges for all complex numbers $z$ except for negative integers in which it fails, as the recursive relationship ${displaystyle m!=m(m-1)!}$ back leads to a division between zero for value ${displaystyle m=0}$ . Post ${displaystyle Gamma (n)=(n-1)!}$ for gamma function, the preceding relationship gives rise to the definition:

{displaystyle Gamma (z)={frac {1}{z}}prod _{n=1}^{infty }{frac {left(1+{frac {1}{n}}right)^{z}}{1+{frac {z}{n}}}},}

valid for non-negative integers.

Weierstrass definition

The definition of gamma function due to Weierstrass is valid for all complex numbers $z$ except for non-positive integer values

{displaystyle Gamma (z)={frac {e^{-gamma z}}{z}}prod _{n=1}^{infty }left(1+{frac {z}{n}}right)^{-1}e^{z/n}}

where $gamma$ is the constant of Euler-Mascheroni.

In terms of generalized Laguerre polynomials

A representation of the incomplete gamma function in terms of the generalized Laguerre polynomials is

{displaystyle Gamma (z,x)=x^{z}e^{-x}sum _{n=0}^{infty }{frac {L_{n}^{(z)(x)}}{n+1}}}

which converges for $-1}" xmlns="http://www.w3.org/1998/Math/MathML">Re(z)▪− − 1{displaystyle {text{Re}}(z) 2005-1}-1}" aria-hidden="true" class="mwe-math-fallback-image-inline" src="https://wikimedia.org/api/rest_v1/media/math/render/svg/e94ea5a9f24529f0cec3a34981b8ce5864491f1d" style="vertical-align: -0.838ex; width:11.709ex; height:2.843ex;"/>$ and $0}" xmlns="http://www.w3.org/1998/Math/MathML">x▪0{displaystyle x 2005}0" aria-hidden="true" class="mwe-math-fallback-image-inline" src="https://wikimedia.org/api/rest_v1/media/math/render/svg/80d24be5f0eb4a9173da6038badc8659546021d0" style="vertical-align: -0.338ex; width:5.591ex; height:2.176ex;"/>$ .

Properties

General

Other important functional equations of the gamma function are Euler's reflection formula

{displaystyle Gamma (1-z);Gamma (z)={pi over operatorname {sen} {(pi z)}},quad znotin mathbb {Z} }

what it implies

{displaystyle Gamma (varepsilon -n)=(-1)^{n-1}{frac {Gamma (-varepsilon)Gamma (1+varepsilon)}{Gamma (n+1-varepsilon)}}}

and Legendre's doubling formula

{displaystyle Gamma (z);Gamma left(z+{frac {1}{2}}right)=2^{1-2z};{sqrt {pi }};Gamma (2z).,!}

The doubling formula is a special case of the multiplication theorem

{displaystyle {begin{aligned}prod _{k=0}^{m-1}Gamma left(z+{frac {k}{m}}right)&=Gamma (z);Gamma left(z+{frac {1}{m}}right);Gamma left(z+{frac {2}{m}}right)cdots Gamma left(z+{frac {m-1}{m}}right)\&=(2pi)^{frac {m-1}{2}}m^{{frac {1}{2}}-mz};Gamma (mz).end{aligned}}}

A basic but very useful property of the gamma function, which can be obtained from the definition in terms of a limit is

{displaystyle {overline {Gamma (z)}}=Gamma ({overline {z}})Longrightarrow Gamma (z)Gamma ({overline {z}})in mathbb {R} }

in particular, ${displaystyle z=a+bi}$ , this product is

{displaystyle left|Gamma (a+bi)right|^{2}=left|Gamma (a)right|^{2}prod _{k=0}^{infty }{frac {1}{1+{frac {b^{2}}{(a+k)^{2}}}}}}

if the real part is an integer, this is ${displaystyle ain mathbb {Z} }$ then.

{displaystyle {begin{aligned}left|Gamma (bi)right|^{2}&={frac {pi }{b;{text{senh}}(pi b)}}\left|Gamma left({frac {1}{2}}+biright)right|^{2}&={frac {pi }{cosh(pi b)}}\left|Gamma (1+bi)right|^{2}&={frac {pi b}{{text{senh}}(pi b)}}\left|Gamma (1+n+bi)right|^{2}&={frac {pi b}{{text{senh}}(pi b)}}prod _{k=1}^{n}(k^{2}+b^{2})\left|Gamma (-nbi)right|^{2}&={frac {pi }{b;{text{senh}}(pi b)}}prod _{k=1}^{n}{frac {1}{k^{2}+b^{2}}}\end{aligned}}}

being ${displaystyle nin mathbb {N} }$ .

Several useful limits for asymptotic approximations:

{displaystyle lim _{nto infty }{frac {Gamma (n+alpha)}{Gamma (n)n^{alpha }}}=1,quad lim _{nto infty }{frac {Gamma (n-alpha)Gamma (n+alpha)}{Gamma (n-beta)Gamma (n+beta)}}=1;qquad alphabeta in mathbb {R} }

Perhaps the best known value of the gamma function with non-integer argument is:

{displaystyle Gamma left({frac {1}{2}}right)={sqrt {pi }},,!}

Which can be obtained by doing ${displaystyle z=1/2}$ in the formula of reflection or in the formula of duplication, using the relationship of the gamma function with the beta function given below with ${displaystyle x=y=1/2}$ or by substitution ${displaystyle u={sqrt {t}}}$ in the integral definition of gamma function, with what is obtained a Gaussiana integral. In general, for non-negative values $n$ you have:

{displaystyle {begin{aligned}Gamma left({frac {1}{2}}+nright)&={frac {(2n)!}{4^{n}n!}}{sqrt {pi }}={frac {(2n-1)!!}{2^{n}}}{sqrt {pi }}={binom {n-{frac {1}{2}}}{n}}n!{sqrt {pi }}\Gamma left({frac {1}{2}}-nright)&={frac {(-4)^{n}n!}{(2n)!}}{sqrt {pi }}={frac {(-2)^{n}}{(2n-1)!!}}{sqrt {pi }}end{aligned}}}

where ${displaystyle n!!}$ denotes to double factorial $n$ .

Derivative

The derivatives of the gamma function are given by the polygamma function, for example:

{displaystyle Gamma '(z)=Gamma (z)psi _{0}(z).}

For a positive integer $m$ , the derivative of the gamma function can be calculated as follows

{displaystyle Gamma '(m+1)=m!left(-gamma +sum _{k=1}^{m}{frac {1}{k}}right)}

where $gamma$ denotes the constant of Euler-Mascheroni.

From the integral representation of the gamma function, it is obtained that $n$ -the figure derived from gamma function is given by:

{displaystyle {frac {d^{n}}{dx^{n}}},Gamma (x)=int _{0}^{infty }t^{x-1}e^{-t}(ln t)^{n}dt}

Waste

The gamma function has an order 1 pole in ${displaystyle z=-n}$ for all non-negative integers. The residue at each pole is:

{displaystyle operatorname {Res} (Gamma-n)={frac {(-1)^{n}}{n!}}.,!}

The Bohr-Mollerup theorem says that, among all the functions that generalize the factorial of the natural numbers to the real numbers, only the gamma function is logarithmically convex, that is, the natural logarithm of the gamma function is a convex function.

Representation as an integral

There are many formulas, besides the of second type Eulerto represent the gamma function as an integral. When the real part of $z$ It's positive then.

{displaystyle Gamma (z)=int _{0}^{1}left(ln {frac {1}{t}}right)^{z-1}dt}

When the real part of ${displaystyle z}$ is positive then the first comprehensive Binet formula for gamma function is

{displaystyle ln Gamma (z)=left(z-{frac {1}{2}}right)ln z-z+{frac {1}{2}}ln(2pi)+int _{0}^{infty }left({frac {1}{2}}-{frac {1}{t}}+{frac {1}{e^{t}-1}}right){frac {e^{-tz}}{t}};dt}

the integral on the right can be interpreted as the Laplace Transform, that is

{displaystyle ln left(Gamma (z)left({frac {e}{z}}right)^{z}{sqrt {2pi z}}right)={mathcal {L}}left({frac {1}{2t}}-{frac {1}{t^{2}}}+{frac {1}{t(e^{t}-1)}}right)(z)}

When the real part of ${displaystyle z}$ is positive then the second comprehensive Binet formula for gamma function is

{displaystyle ln Gamma (z)=left(z-{frac {1}{2}}right)ln z-z+{frac {1}{2}}ln(2pi)+2int _{0}^{infty }{frac {arctan left({frac {t}{z}}right)}{e^{2pi t}-1}};dt}

Fourier series expansion

The logarithm of the gamma function has the following development in Fourier series for $<math alttext="{displaystyle 0<z0.z.1{displaystyle 0 ingredient1} <img alt="{displaystyle 0<z$ :

{displaystyle ln Gamma (z)=left({frac {1}{2}}-zright)(gamma +ln 2)+(1-z)ln pi -{frac {ln operatorname {sen}(pi z)}{2}}+{frac {1}{pi }}sum _{n=1}^{infty }{frac {ln n}{n}}operatorname {sen}(2pi nz)}

which for a long time was attributed to Ernst Kummer who proved it in 1847. However, it was discovered that Carl Johan Malmsten proved it for the first time in 1842.

Raabe's formula

In 1840, Joseph Ludwig Raabe showed that

{displaystyle int _{a}^{a+1}ln Gamma (z)dz={frac {ln(2pi)}{2}}+aln a-a}

for values $0}" xmlns="http://www.w3.org/1998/Math/MathML">a▪0{displaystyle a vocabulary0}0}" aria-hidden="true" class="mwe-math-fallback-image-inline" src="https://wikimedia.org/api/rest_v1/media/math/render/svg/1f34a80ea013edb56e340b19550430a8b6dfd7b9" style="vertical-align: -0.338ex; width:5.491ex; height:2.176ex;"/>$ .

In particular, when ${displaystyle a=0}$ We get

{displaystyle int _{0}^{1}ln Gamma (z)dz={frac {ln(2pi)}{2}}}

Pi function

Gauss introduced an alternative notation for the gamma function called the Pi function, which in terms of the gamma function is:

{displaystyle Pi (z)=Gamma (z+1)=z;Gamma (z)=int _{0}^{infty }e^{-t}t^{z}dt}

Thus, the relationship of the Pi function with the factorial is more natural than in the case of gamma function as for any non-negative integer $n$

{displaystyle Pi (n)=n!}

The reflection formula takes the following form:

{displaystyle Pi (z);Pi (-z)={frac {pi z}{operatorname {sen}(pi z)}}={frac {1}{operatorname {sinc} (z)}}}

Where ${displaystyle {text{sinc}}}$ is the standardized sinc function, while the theorem of multiplication takes the form:

{displaystyle Pi left({frac {z}{m}}right),Pi left({frac {z-1}{m}}right)cdots Pi left({frac {z-m+1}{m}}right)=left({frac {(2pi)^{m}}{2pi m}}right)^{1/2},m^{-z},Pi (z).,!}

Sometimes the following definition is found

{displaystyle pi (z)={frac {1}{Pi (z)}},,!}

where ${displaystyle pi (z)}$ is an entirely defined function for any complex number, since it has no poles. The reason for this is that the gamma function and therefore the Pi function have no zeros.

Relationship with other functions

In the integral representation of the gamma function, both the upper and lower limit of the integration are fixed. The superior incomplete gamma function ${displaystyle gamma (a,x)}$ lower ${displaystyle Gamma (a,x)}$ are obtained by modifying the upper or lower integration limits respectively.

{displaystyle Gamma (a,x)=int _{x}^{infty }t^{a-1},e^{-t},dt.,!}

{displaystyle gamma (a,x)=int _{0}^{x}t^{a-1},e^{-t},dt.,!}

The gamma function is related to the beta function by the following formula

{displaystyle mathrm {B} (x,y)={frac {Gamma (x);Gamma (y)}{Gamma (x+y)}}.,!}

The logarithmic derivative of the gamma function is the digomma function ${displaystyle psi ^{(0)}(z)}$ . Major derivatives are polygamma functions ${displaystyle psi ^{(n)}(z)}$ .

{displaystyle psi (x)=psi ^{0}(x)={frac {Gamma '(x)}{Gamma (x)}}}

{displaystyle psi ^{(n)}(x)=left({frac {d}{dx}}right)^{n}psi (x)=left({frac {d}{dx}}right)^{n+1}log Gamma (x)}

The analog of the gamma function on a finite body or a finite ring are the Gaussian sums, a type of exponential sum.
The reverse gamma function is the reverse of the gamma function, which is an entire function.
The gamma function appears in the integral definition of Riemann's zeta function ${displaystyle zeta (z)}$ :

{displaystyle zeta (z)={frac {1}{Gamma (z)}}int _{0}^{infty }{frac {u^{z-1}}{e^{u}-1}};mathrm {d} u,!.}

Valid formula only if $1}" xmlns="http://www.w3.org/1998/Math/MathML">Re (z)▪1{displaystyle operatorname {Re} (z) 2005}1}" aria-hidden="true" class="mwe-math-fallback-image-inline" src="https://wikimedia.org/api/rest_v1/media/math/render/svg/56497bbfa5e1795edfdbc22a6f0c52b271f70bfd" style="vertical-align: -0.838ex; width:9.901ex; height:2.843ex;"/>$ . It also appears in the functional equation ${displaystyle zeta (z)}$ :

{displaystyle pi ^{-z/2};Gamma left({frac {z}{2}}right)zeta (z)=pi ^{-{frac {1-z}{2}}};Gamma left({frac {1-z}{2}}right);zeta (1-z).}

Particular values

Some particular values of the gamma function are

{displaystyle {begin{aligned}Gamma left(-{frac {3}{2}}right)&={frac {4{sqrt {pi }}}{3}}&approx & 2,363\Gamma left(-{frac {1}{2}}right)&=-2{sqrt {pi }}&approx &-3,545\Gamma left({frac {1}{2}}right)&={sqrt {pi }}&approx & 1,772\Gamma (1)&=0!&=& 1\Gamma left({frac {3}{2}}right)&={frac {sqrt {pi }}{2}}&approx & 0,886\Gamma (2)&=1!&=& 1\Gamma left({frac {5}{2}}right)&={frac {3{sqrt {pi }}}{4}}&approx & 1,329\Gamma (3)&=2!&=& 2\Gamma left({frac {7}{2}}right)&={frac {15{sqrt {pi }}}{8}}&approx & 3,323\Gamma (4)&=3!&=& 6\end{aligned}}}

Approximations

The gamma function can be calculated numerically with arbitrary precision using the Stirling formula, the Lanczos approximation, or the Spouge approximation.

For arguments that are integer multiples of 1/24, the gamma function can be quickly evaluated using iterations of arithmetic geometric means (see Values of the gamma function).

Because both the gamma function and the factorial grow very rapidly for moderately large arguments, many computer programs include functions that return the logarithm of the gamma function. This grows more slowly, and in combinatorial calculations it is very useful, since you go from multiplying and dividing large values to adding or subtracting their logarithms.

Applications of the gamma function

Fraction Calculation

Main article: Fractional calculation

La $n$ -thousand derived from ${displaystyle ax^{b}}$ (where n is a natural number) can be seen as follows:

{displaystyle {frac {d^{n}}{dx^{n}}}left(ax^{b}right)=left(b-n+1right)cdots left(b-2right)left(b-1right)bax^{b-n}={frac {b!}{left(b-nright)!}}ax^{b-n}}

Like ${displaystyle n!=Gamma (n+1)}$ then.

{displaystyle {frac {d^{n}}{dx^{n}}}left(ax^{b}right)={frac {Gamma left(b+1right)}{Gamma left(b-n+1right)}}ax^{b-n}}

where $n$ can be any number where gamma is defined or can be defined by limits. This way you can calculate for example the 1/2 derivative $x$ Of $x^{2}$ and even a constant ${displaystyle c=cx^{0}}$ :

{displaystyle {frac {d^{frac {1}{2}}}{dx^{frac {1}{2}}}}left(xright)={frac {2{sqrt {x}}}{sqrt {pi }}}}

{displaystyle {frac {d^{frac {1}{2}}}{dx^{frac {1}{2}}}}left(x^{2}right)={frac {8{sqrt {x^{3}}}}{3{sqrt {pi }}}}}

{displaystyle {frac {d^{frac {1}{2}}}{dx^{frac {1}{2}}}}left(cright)={frac {c}{{sqrt {pi }}{sqrt {x}}}}}

Contenido relacionado

Más resultados...