Fullerene
A fullerene (also, fullerene) is a molecule composed of carbon that can adopt a geometric shape reminiscent of a sphere, an ellipsoid, a tube (called a nanotube) or a ring. Fullerenes are similar to graphite, composed of sheets of linked hexagonal rings, but containing pentagonal and sometimes heptagonal rings, which prevents the sheet from being flat. Fullerenes are the third known stable molecular form of carbon, after graphite and diamond.
Fullerenes were discovered in 1985 by Harold Kroto, Robert Curl and Richard Smalley, which earned them the Nobel Prize in Chemistry in 1996.
The first fullerene discovered was C
60, consisting of 12 pentagons and 20 hexagons. Each vertex corresponds to a carbon atom and each edge to a covalent bond. It has a structure identical to the geodesic dome or a soccer ball. For this reason, it is called "buckminsterfullerene" (in homage to the architect Buckminster Fuller who designed the geodesic dome) or "football". Spherical fullerenes are often called buckyspheres and cylindrical fullerenes are often called buckytubes or nanotubes.
They stand out for their versatility for the synthesis of new compounds. Its nature and form have become widely known in science and in culture in general, due to its physical, chemical, mathematical and aesthetic characteristics.
Buckminsterfullerene or fullerene of C60
The best known fullerene is the one formed by 60 carbon atoms (C60), in which none of the pentagons that compose it share an edge; if the pentagons have an edge in common, the structure will be destabilized (see pentalene). The structure of C60 is that of a truncated icosahedron, which resembles a soccer ball whose design began with the Telstar 1970. It is made up of 20 hexagons and 12 pentagons, with one carbon atom at each of the vertices of the hexagons and a link along each edge. Although its name comes from Richard Buckminster Fuller for his geodesic domes -the first from 1948-, it was the German engineer Walther Bauersfeld who in 1912 began the construction of a work with that shape for the Carl Zeiss optical instrument company, in Jena. The oldest known drawing of the truncated icosahedron is that of Piero della Francesca and the best known is that Leonardo da Vinci made for the book The Divine Proportion commissioned by Luca Pacioli.
Other fullerenes
The fullerene C20 is the smallest of all, it has no hexagons, only 12 pentagons forming a dodecahedron, while the C70, has 12 pentagons the same than buckminsterfullerene, but it has more hexagons, and its shape in this case resembles a rugby ball.
A nanotube is a structure formed by polymerized cylindrical fullerenes, in which the carbon atoms from a certain point bond with the carbon atoms of the next fullerene. These structures present very usable properties for the industry such as electrical permittivity. The strength between the links makes it a very solid material and resistant to high temperatures. In addition, it can be doped with other materials to change its properties, such as ductility or bond strength, which would allow the creation, for example, of a new generation of low-cost electronic chips.
Production and discovery
Until the 20th century, graphite and diamond were the only known allotropes of carbon. In molecular spectroscopy experiments, peaks were observed that corresponded to molecules with an exact molecular mass of 60, 70, or more carbon atoms. Harold Kroto of the University of Sussex, James Heath, Sean O'Brien, Robert Curl and Richard Smalley of Rice University discovered C60 and other fullerenes in 1985, in a experiment that consisted of making a laser beam affect a piece of graphite. They did expect to discover new allotropes of carbon, but they assumed they would be long molecules, rather than the spherical and cylindrical shapes they found. Kroto, Curl and Smalley were awarded the Nobel Prize in Chemistry in 1996 for their collaboration in the discovery of this class of compounds. C60 and other fullerenes were later observed outside the laboratory (eg in candle soot). By 1991, it was relatively easy to produce a few grams of fullerene powder using the techniques of Donald Huffman and Wolfgang Krätschmer. The purification of fullerene was a challenge for chemists until the first decade of this century, when a team of Spanish researchers developed a new process for obtaining it. The endohedral fullerenes have incorporated, between the atoms of the lattice, ions or other smaller molecules. Fullerene is a common reagent in many organic reactions, such as the Bingel reaction, discovered in 1993.
Fullerenes in space
In July 2010 NASA announced the discovery of fullerenes in space. Using the sensitive infrared vision of the Spitzer telescope, the researchers have confirmed the presence of C70 in the planetary nebula Tc1. Astronomers believe that fullerenes are created in the outer layers of a star, such as our Sun, and are subsequently ejected into space after the star's explosion.
Properties
Since their discovery, the chemical and physical properties of fullerenes are still under intense study. Among the most relevant physical properties is the energy gap between the highest energy occupied orbital (HOMO) and the lowest energy unoccupied orbital (LUMO), whose measure is ca. 1.7 eV. The ground state symmetry of C60 fullerene corresponds to point group Ih. In this symmetry the HOMO and LUMO orbitals are five and three times degenerate, hu and t1u respectively. Due to this fact, electronic transitions from HOMO to LUMO are symmetry forbidden. C60 fullerene exhibits 174 normal modes of vibration (3N - 6, where N = 60 carbon atoms) in the infrared region. However, only four normal modes are active.
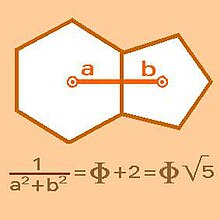
In the truncated icosahedron and the geometric bodies similar to it —the hexapentas— the mathematical key lies in the geometric relationship between the hexagons and the pentagons that make them up. The demonstration of the harmony in the hexapentas in general, given the consonance between their component hexagons and pentagons (considering that both figures in each case have the same length of their sides or edges of the polyhedron), is given in the relation of their apothems by means of the Golden Ratio (ф = 1,618…).
In the field of nanomedicine, C60 fullerene has been studied for its potential medicinal use as a fixative for specific antibiotics in its structure to attack resistant bacteria and certain cancer cells, such as melanoma.
Fullerenes are not very reactive due to the stability of graphite-like bonds, and they are also very slightly soluble in most solvents. Common solvents for fullerenes include toluene and carbon disulfide. Solutions of pure buckminsterfullerene have a deep purple color. Fullerene is the only allotrope of carbon that can be dissolved. Researchers have been able to increase their reactivity by attaching active groups to the surfaces of fullerenes. Buckminsterfullerene does not exhibit "superaromaticity", that is, the electrons in the hexagonal rings cannot delocalize in the entire molecule.
Other atoms can be trapped inside fullerenes; In fact, there is evidence of this thanks to the analysis of the noble gas preserved in these conditions after the impact of a meteorite at the end of the Permian period. In the field of nanotechnology, thermal resistance and superconductivity are some of the most deeply studied characteristics.
A common method of producing fullerenes is to pass a strong electric current between two nearby graphite electrodes in an inert atmosphere. The resulting arc between the two electrodes produces a soot deposit from which many different fullerenes can be isolated.
Potential risks
Although buckyspheres are thought to be relatively inert in theory, a presentation given to the American Chemical Society in March 2004 and described in an article published in New Scientist on April 3, 2004, suggests that the molecule is harmful to organisms. An experiment carried out by Eva Oberdörster at Southern Methodist University, in which she introduced fullerenes into water at concentrations of 0.5 parts per million, showed that a black bass fish (Micropterus salmoides ) suffered 17 times more cell damage in brain tissue 48 hours later. The damage consisted of lipid peroxidation at the level of the cell membrane, which impairs its functioning. There was also inflammation in the liver and the activation of genes related to the synthesis of repair enzymes.
Solubility
The following list shows the solvents in order of decreasing solubility for a C60/C70 mixture). The values in parentheses indicate the saturation concentration.
- 1.2.4-trichlorobenzene (20 mg/mL)
- carbon dioxide (12 mg/mL)
- toluene (3.2 mg/mL)
- benzene (1.8 mg/mL)
- chloroform (0.5 mg/mL)
- carbon tetrachloride (0.4 mg/mL)
- cyclehexane (0.054 mg/mL)
- n-hexane (0.046 mg/mL)
- tetrahydrofuran (0.037 mg/mL)
- acetonitrile (0.02 mg/mL)
- methanel (0,0009 mg/mL)
Maths of Fullerenes
In mathematical terms, the structure of a fullerene is a convex polyhedron with pentagonal and hexagonal faces.
With the help of Euler's formula: faces + vertices - edges = 2, in addition to the fact that each vertex in a fullerene structure belongs to exactly three faces, it can be easily shown that there are exactly 12 pentagons in a fullerene. The smallest fullerene is C20, the dodecahedron. There are no fullerenes with 22 vertices. The number of different C2n fullerenes grows very rapidly with increasing value of n; for example, there are 1812 C60 fullerenes, but only one of them, buckminsterfullerene, has no adjacent pentagons.
Buckminsterfullerene in the plastic arts
Physicist turned artist Julian Voss-Andreae has created a number of sculptures symbolizing the wave-particle duality in buckminsterfullerenes. Voss-Andreae was involved in research to show that objects as large as buckminsterfullerenes also obey the peculiar laws of quantum physics. After this, Voss-Andreae decided to change his profession to become a full-time artist. Since then he has designed objects such as the 24-inch-diameter bronze structure called the "Quantum Buckysphere"; (2004) consisting of four nested buckyspheres. His largest sculpture based on fullerenes is located in a private park in Portland, Oregon (United States). Quantum Reality (Great Buckysphere Surrounded by Trees) (2007) is a 9 meter diameter steel structure pierced by several trees that grow freely in the middle of it and hold it in the air just above the ground. arms reach.
In-depth readings
- The Most Beautiful Molecule: The Discovery of the Buckyball by Hugh Aldersey-Williams (John Wiley & Sons, 1995) ISBN 0-471-19333-X
Contenido relacionado
Sodium carbonate
Alkynyl group
Calcium carbonate