Complementary angles

The α and β angles are complementary.

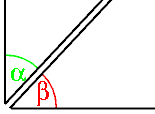
The complementary angles are those angles whose measures add up to 90 sexagesimal degrees, that is, if two complementary angles are in turn consecutive, their non-common sides form a right angle. Vbc 10 10

Examples

• The complementary angle of α α {displaystyle alpha }technically α α {displaystyle alpha } a range of 70 {displaystyle 70^{circ}}It'll be an angle. β β {displaystyle beta } with a width equal to the difference between α α {displaystyle alpha } and 90 {displaystyle 90^{circ}}:

β β =90 − − 70 =20 {displaystyle beta =90^{circ }-70^{circ}=20^{circ }}}

therefore the angle β β {displaystyle beta } is the complement of α α {displaystyle alpha } since α α +β β =90 {displaystyle alpha +beta =90^{circ}}}.

• The triangle rectangle has two complementary angles since the triangle being has to 180 =90 +α α +β β {displaystyle 180^{circ }=90^{circ }+alpha +beta } therefore 90 =α α +β β {displaystyle 90^{circ}=alpha +beta }.

• The diagonal of a rectangle also sets complementary angles with adjacent sides.